Personalised Dosing of Hyperthermia

Hyperthermia as an oncologic therapy has a long history. Although the treatment has a long history; hyperthermia has only recently become accepted as a valid option. Sceptical opinions in connection with hyperthermia dominate the clinical practice, and the sometimes "miraculous” results of hyperthermia raises the scepticism even higher; the “miracles” are naturally out of the realm of our current scientific approaches.

The goal of hyperthermia in oncology is, of course, to eliminate malignant cells. The tool has the thermal effect, which could be provided by various kinds of energy absorptions [1]. It is considered a complementary therapy. Its clinical applications mostly concentrate on various chemo- and radiotherapies allowing the physiological feedback to support these therapies through heat flow and intensified blood flow. This, in turn, affects drug delivery and oxygenation in chemo- and radiotherapies [2].

We know from everyday practice that the difference between poison and medicine is merely the dose. Dose is an important factor for efficacy, safety and reproducibility, too [3]. In the case of medication or radiation oncology, we know dose units as quantitatively measurable values in mg/m2 or J/kg in chemo- or radiotherapies, respectively. The main challenge in the clinical use of hyperthermia is the lack of a definite dose concept; consequently, the repeatability of a given therapy gives way to serious doubts.

In hyperthermia, temperature is overemphasized as a dose; since it is not a quantitative parameter. Rather, it is a quality that creates equilibrium spread in the system. In chemotherapy, cytotoxic remedies could have very serious side effects, and the role of their safety has been emphasized. Chemotherapy doses are determined by safety (toxicity) limits, independently of the individual person or the size of the tumorous target. The result (efficacy) is measured a definite time later, when it is measurable or symptoms of toxicity (by personal variability) appear. Then, the chemo dose is modified or a complete change of medication is applied. The actual dose varies then, considering the actual patient and the specific situation.

When the medication demonstrates no side effects (or the side effects are controlled) in the individual, then the dose, according to the safety role, has no upper limit. When the dose is limited but it is too high for the patient due to the biovariable poisoning limit, then the actual applied dose is lowered according to the needs of the particular patient.

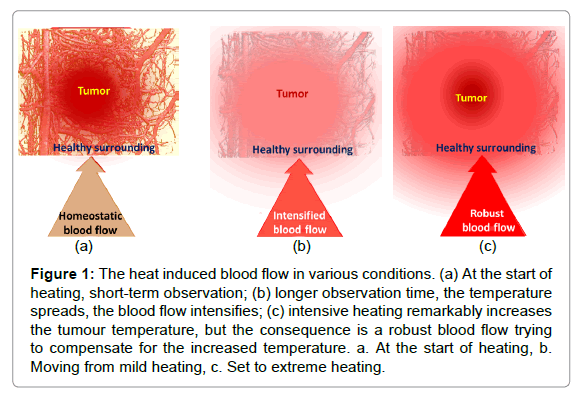
The dose concept, which is applied in ionising radiation (Gy), causes problems in non-ionising cases: the provided energy naturally spreads despite the careful focusing of the beam. Applying a certainly local, invasive heating (ablation), the time of heating is short; the provided specific energy could characterise the process. However, in the case of non-invasive local applications, the physiological feedback (thermal homeostasis) becomes active and spreads the heat during the relatively long treatment time. After this longer time, the thermal homeostatic control becomes active and vasodilates the arteries to maintain homeostasis. The characteristic reaction time of the blood flow (washout time) is approx. five to seven minutes in humans [4], which is the threshold of using the absorbed energy as a controlling dose. Over this limit, thermal homeostasis is active; the actual heat exchange of the target with its environment determines the actual heating process (Figure 1). The intensive blood flow could increase tumour growth, as well as the risk of metastases, suppressing the possible curative effect.

Figure 1: The heat induced blood flow in various conditions. (a) At the start of heating, short-term observation; (b) longer observation time, the temperature spreads, the blood flow intensifies; (c) intensive heating remarkably increases the tumour temperature, but the consequence is a robust blood flow trying to compensate for the increased temperature. a. At the start of heating, b. Moving from mild heating, c. Set to extreme heating.

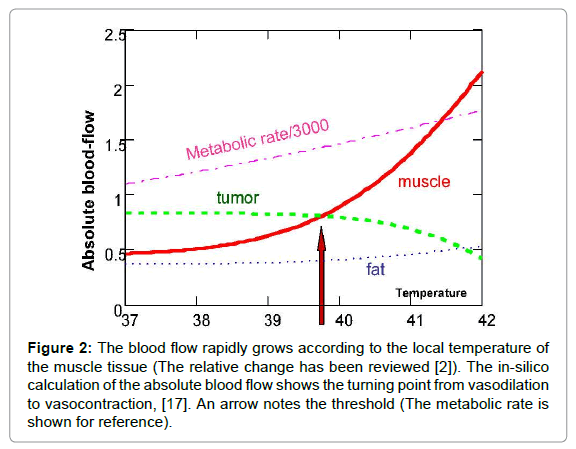
However, like everything in the complex networks of negatively feedback controls, the high blood flow can have the opposite effect, too – the high blood flow delivers more chemo-drugs and sensitises the individual to radiotherapy, as well. When forcing higher temperatures on the tumour, there is another effect on the blood supply, which was pointed out first by Song [5,6] and later by others [7-10]. This suppressed blood flow, and consequently the limited heat spreading, create another situation [11-19]. A calculation showed the absolute blood flow changes, defining the threshold in silico [20], where the blood flow of surrounding muscles overtakes the tumours (Figure 2). These considerations opened a new approach to hyperthermia, pointing out the importance of physiological feedback mechanisms that do not naturally exist in vitro and could vary by species in vivo, and by individual in clinical applications. The blood vessels of the tumour sustain vasocontraction over a temperature threshold. This threshold depends on many actual factors, but ranges between 39 and 42°C.

Figure 2: The blood flow rapidly grows according to the local temperature of the muscle tissue (The relative change has been reviewed [2]). The in-silico calculation of the absolute blood flow shows the turning point from vasodilation to vasocontraction, [17]. An arrow notes the threshold (The metabolic rate is shown for reference).

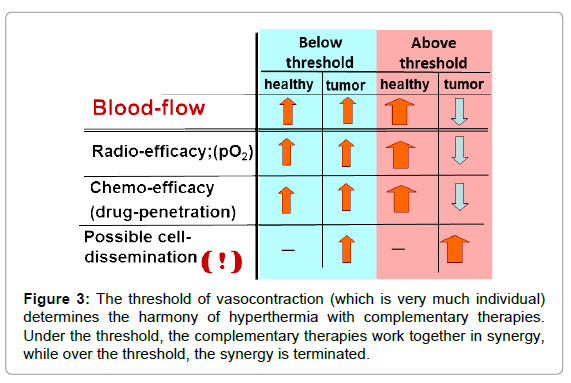
Vasocontraction functions as a heat trap [21] for the tumour and helps its local heating by increasing the temperature rapidly in the tumour compared to the non-tumorous regions. This is an “apparent” success. It looks like a quick and effective heating, but in fact, the complementary therapies are blocked (Figure 3).

Figure 3: The threshold of vasocontraction (which is very much individual) determines the harmony of hyperthermia with complementary therapies. Under the threshold, the complementary therapies work together in synergy, while over the threshold, the synergy is terminated.

Together with this blockage, the periphery (which is the most vivid part of the tumour) has intensive blood flow and rapidly increases the risk of invasion and dissemination. This could be the reason why local control is sometimes miraculously successful, while the overall survival [22,23] and toxicity [24] levels tend towards the opposite.

When the goal is the reproducibility of the treatment, all of the above parameters have to be controlled. The main parameter to check and regulate is the vasocontraction threshold, which could essentially modify the complete protocol of the therapy.

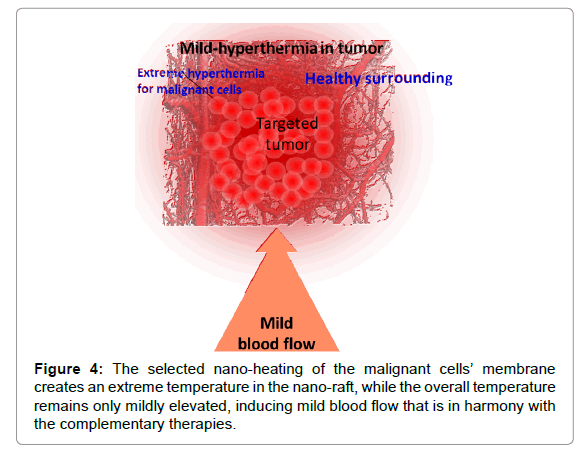
The solution must be complex, like the situation itself: we have to heat up the malignant cells to an extreme level without igniting robust blood flow as feedback to compensate for the thermal misbalance. This issue is addressed when selecting and heating the malignant cells. Their mass is much less compared to the complete tumour itself, so the heating energy is also relatively small. The heat naturally spreads over time, but it causes only mild hyperthermia in the tumour at the time when the malignant cells are heated more intensively (Figure 4). It is highly probable that the mass temperature of the tumour does not exceed the vasocontraction threshold. Consequently, the complementary clinical applications are stable during the therapy. This method is referred to as oncothermia, and information on this particular method has been published elsewhere [25,26].

Figure 4: The selected nano-heating of the malignant cells’ membrane creates an extreme temperature in the nano-raft, while the overall temperature remains only mildly elevated, inducing mild blood flow that is in harmony with the complementary therapies.

By its very definition, hyperthermia is a thermal process. The Arrhenius plot could be regarded as proof of the thermal character when the reaction rate exponentially depends on the inverse temperature. This probability distribution is the basis of simple chemical kinetics and determines the Arrhenius equation [27,28]:

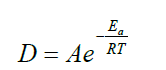(1)

Where E_a is the activation energy of the given reaction [R is the universal gas constant, R ≈ 8.3 J/K/mol), T is the absolute temperature], A is the pre-exponential (normalising) factor, and D is the rate constant of the given reaction in T temperature.

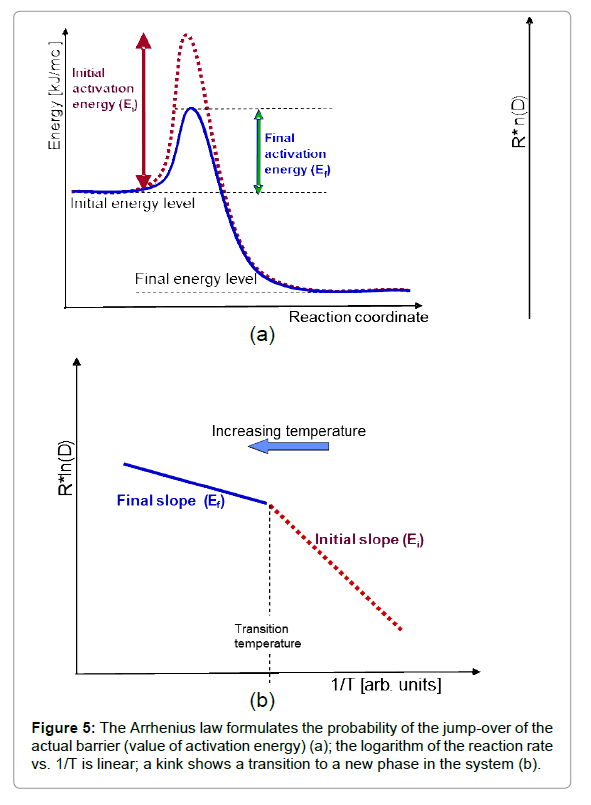
This simple relationship is the consequence of the ratio of activation energy (E_a) to thermal energy (RT), showing the reaction when the thermal energy is large enough to exceed the barrier by E_a. Through the gradually increasing thermal energy, a reaction (going over the barrier E_a) becomes more likely, expressing the exponential probability of the Arrhenius law. The logarithm of the reaction rate vs. the inverse temperature shows a linear dependence in the case of thermal effects, and the value of the slope characterizes the activation energy (Figure 5). When the slope changes, a kink appears showing a phase transition like character when the new phase has new activation energy (new bonding).

Figure 5: The Arrhenius law formulates the probability of the jump-over of the actual barrier (value of activation energy) (a); the logarithm of the reaction rate vs. 1/T is linear; a kink shows a transition to a new phase in the system (b).

However, characteristic non-Arrhenius behaviours could be observed in complex systems [29]. These are multi-step reaction mechanisms or radical changes in the reactions, producing chemical reactions or restructuring the system during the process (e.g. phase transition occurs). The living objects regularly consume the energy in multi-step processes and could be described by the multiple kinks on the actual steps on the Arrhenius plot. The metabolic rate and body temperature are definitely connected having Arrhenius-like behaviour with 0.6–0.8 eV activation energy and a mass dependent pre-exponential factor [30].

The key issue with medical acceptance is the therapy’s protocol, which directly shows the demand for a definition of the dose [31]. The dosing of hyperthermia, however, remains a challenge.

In case of dosing there are three parameters to be considered:

1. The value that is prescribed in the complete individual protocol for the particular patient (repeatability).

2. The value that could be controlled during the treatment process to ensure the proper therapy (process control).

3. The value that is under the tolerance limit of the actual patient when the prescribed dose is administered (safety).

A strong indication from clinical practice is patient tolerance (safety), as this governs the overall therapy. The majority of the treated patients cannot have the prescribed dose due to issues with tolerance [32], and the protocol of the actual treatment is based on the patient’s tolerance [33], the heat increase has to be stopped when the patient experiences remarkable discomfort. Other studies have excluded lowtolerance patients (not-heatable) from the study [34].

Presently, in most researches, hyperthermia uses temperature as the basis of the dose, as well as to determine the safety limit. Unfortunately, the temperature–dose does not satisfy an important requirement of the dosing: the extensive behaviour. Temperature does not depend on any size parameters. To overcome this problem and consider the time dependence of hyperthermia, time and temperature were used in parallel, resulting in doses that consider the length of time that a particular temperature was maintained. This simply creates a unit (temperature multiplied by time, [Ks]) that has no physical relevance.

Using the surprisingly accurate in vitro fits of the Arrhenius plot for the experimental results [35,36], CEM43°CT90 was introduced [37], measuring the cumulative equivalent minutes at 43°C where the temperature exceeds the 43°C at 90% of the locations during treatment (referred to as the thermal isoeffect dose at 90% of the area) [38]. Unfortunately, it is such a complicated construction with a very complex way of measuring that it is not viable in practice. This problem is demonstrated in the case of whole body hyperthermia, where it is very easy to measure this dose (basically, the body and the tumour inside are at a homogenous temperature), but the results are very different from the same dose provided by the local–regional treatments. It is even more interesting that the lower CEM43°CT90 dose applied with local– regional treatment provides better results compared to the increased dose in the whole body treatment. Therefore, we can claim that this dose unit does not satisfy the basic requirements for the dose concept in general [39-42].

The problem is simple compared to the complexity of the human body and in consequence the complexity of its treatments [43]. The real physiological feedback mechanisms drastically modify the in vitro or phantom-measured dose definition. However, measuring the actual physiologic parameters is very complicated, if it is possible at all. Choosing the actual malignant target in the living body is a complex task which needs complex approach too [44,45]. A general indication of the actual situation could be measured via the impedance during the electromagnetic heating processes [46-48]. In addition, the Arrhenius activation energy could be measured using the impedance [49]. Nevertheless, the impedance depends on multiple actual physiologic changes and personal variants, which are thus far not reproducible for use in dosing hyperthermia.

There are two concepts in the heating dynamism: step-down and step-up heating processes. Step-down heating means starting at a high power [50]. The applied high power could be used for short-duration, over long-duration, tolerance of the patient, and it gets down-regulated when the patient complains. The principle behind step-down heating is based on the speciality of the Arrhenius plot for heating the tumour.

The step-down heating intends the phase transition, which is measured using the slope of the Arrhenius plot. When heating the tumour, the activation energy suddenly changes at around 42°C, and remains at this significantly lower value, even when cooling down to the temperature of the kink, where the activation energy was high previously. This is a characteristic of the irreversible phase transition and helps to destroy the cancer cells using lower energy (step-down). This idea is well-proven in vitro, but casts numerous doubts in vivo. It seems that over the phase transition, the cells are necrotising, so further heating at low temperatures is superflouos. Another modification is that the necrotic tissue has no fresh blood perfusion, so a rapid increase in temperature at this local spot is likely. It is apparent, however, that it is unnecessary from the cell destruction point of view.

The kink temperature appears to be accurately reproducible among the identical conditions; however, this could change depending on the actual circumstances.

• The kink of the Arrhenius graph depends on the applied chemotherapy [51,52]. This is important because hyperthermia is complementary in a large number of cases.

• The kink of the Arrhenius graph depends on the prehistory and dynamics of the treatment [53-57].

• The Arrhenius graph gives different time doses for the different points of the target (because of its non-homogeneous structure); this promotes chemical reactions and lowers the activation energy [58].

The physiological feedback and the vasodilatation/vasocontraction threshold also make a difference in step-down heating. The Arrhenius kink, which has to be overheated, corresponds well to the believed cellular phase transition observed at around 42.5°C [59], and the sudden intense heating at the beginning could lead the system over the vasocontraction threshold. In this case, the applied complementary therapies could be considerably suppressed. Consequently, the complementary application of the step-down heating with chemotherapy needs careful consideration. Blocking the blood flow before the chemo had reached its maximum intake in the tumour suppresses the chemo-efficacy, thus reducing the advantage of the complementary application.

Through the induced vasocontraction, the cooling effect of the bloodstream is drastically decreased. In consequence of this low blood supply, much less energy is required to maintain this temperature compared to the situation when the blood significantly cooled the area. The tumour’s blood flow depends on its weight using negative logarithmic function [60], which further promotes a quick rise of the tumour’s temperature. The process is directly connected to the temperature expectations and the actual immediate real time changes in the tumour status. Its real advantage is the relatively low energy supply after overcoming the relatively high activation energy. Step-down heating is a good option for temperature oriented hyperthermia approaches.

Step-up heating uses a different philosophy. While step-down heating focuses on the tumour and its elimination via necrosis, stepup heating concentrates on the patient’s homeostasis in an attempt to be in harmony with the complexity of the body, helping the natural actions’ during the treatment. The viewpoint of step-down heating is good local control with immediate cell-killing (necrosis); the stepup heating considers the integrative patient oriented actions that are in synchrony with the homeostatic equilibrium, causing minimal discomfort to the patient. With this gentle approach, step-up heating focuses on quality of life and survival time instead of local control. This methodology fits well with the new trend towards the personalisation of oncological treatments [61].

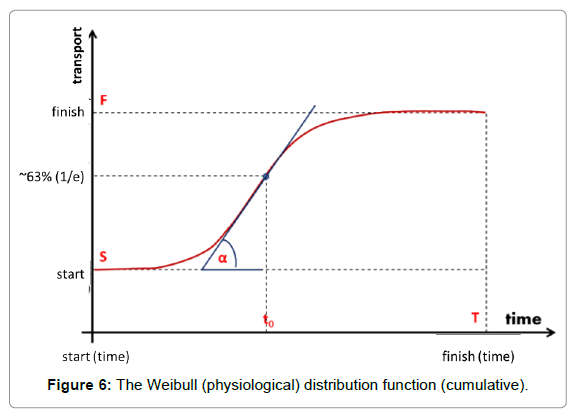
The natural physiological processes form a dynamic equilibrium, dominated by homeostatic logistics of transports in the complex biosystems. The physiological logistic distribution function is formally identical with the typical general logistics and it is the Weibull distribution [62]:

(2)

Where t_o is the unit time when the value of the function is 1/e≈0.63 and the a-power in the distribution defines the shape of the curve (Figure 6). The derivative in the inflexion point equals (a/t_o)·[≈0.63*a, when t_o=1]. The popular interpretation of the parameters is: t_o is the stretching in x-direction (time–transformation) and a is the stretching in y (incline of the curve).

Figure 6: The Weibull (physiological) distribution function (cumulative).

The a-exponents, which are strictly connected to the α-slope, were measured in various bio-transport processes. Cope [63,64] functionally studied the so-called Avrami-exponents (a parameter I Weibull distribution), showing the universality of this logistic function.

The Weibull distribution function approaches multiple clinical applications and is well-established, both theoretically and practically [65-68]. It is used for survival studies in gerontology [69,70] and in oncology [71]. The Weibull distribution could be approached using a normal (Gaussian) distribution over a>2. Step-up heating follows the Weibull function for the best homeostatic support.

A further advantage of the step-up heating process is the selective manipulation of the development of heat-shock proteins (HSPs). A portion of the HSPs rapidly appears during heating [72]. Both the malignant and healthy cells develop HSPs, but their amount is significantly different, [73]. The stressed malignant cells develop fewer than 50% more HSPs compared to their normal high value, while in healthy cells, the stress is “new”, and thus they develop approx. eight times as many HSPs compared to the level prior to the stress. At the end of the process the amount of HSPs is approximately equal in both cell types [67]. This has a great selection advantage – the step-up process could produce better heat tolerance compared to the malignant cells, but the development of this difference needs time, which the stepup heating process allows. The radiative (phase-array) hyperthermia treatments started with step-down heating [32], which later was changed to a step-up process [30].

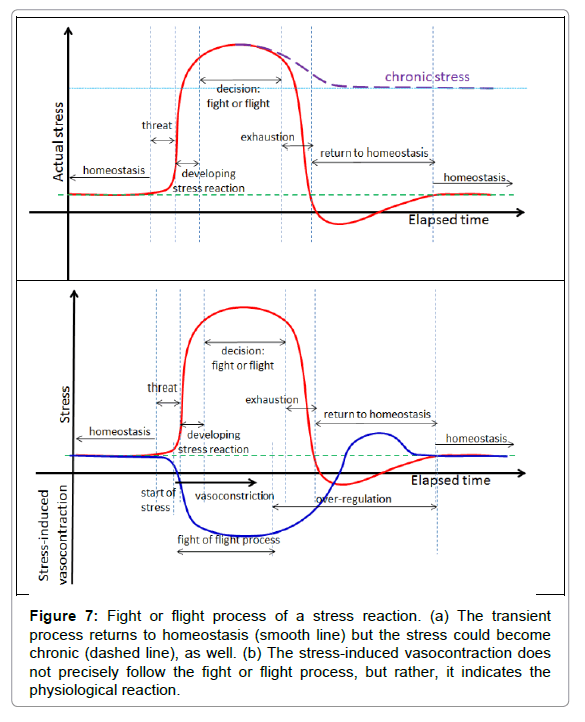
The stress for the patient from the treatment process itself is also an important factor in sensing the tolerance and adjusting the actual dose. Stress is a personalised response, but it is consensual, so in principle it is ideal for a dosing frame. Treatment stress induces the sympathetic nervous system to kick-out the complex living object from its actual homeostatic state activation using the parasympathetic network for negative feedback corrections [74]. This effect is more complex than physiology itself; this is psycho-physiology [75], modifications of the fight or flight decision–response process depend on the actual psychology status of the individual [76,77] (Figure 7).

Figure 7: Fight or flight process of a stress reaction. (a) The transient process returns to homeostasis (smooth line) but the stress could become chronic (dashed line), as well. (b) The stress-induced vasocontraction does not precisely follow the fight or flight process, but rather, it indicates the physiological reaction.

The fight or flight response activates and reorients the energy in the living system to concentrate on a possible emergency situation. It makes important physiologic rearrangements by increasing the blood supply of the prime organs through vasodilatation, it pumps up their metabolic flux, and makes a parallel decrease in the metabolic rate in other parts of the system, mainly via vasocontraction. In the oncothermia application, an important consequence is the decrease of blood flow in the skin. In case of transient stress, the feedback seeks the system’s homeostasis again, and during this period the cutaneous volume has high blood perfusion and sweating could occur. This is the consequence of radiation of the extra heat produced by increased metabolism of the prime organs.

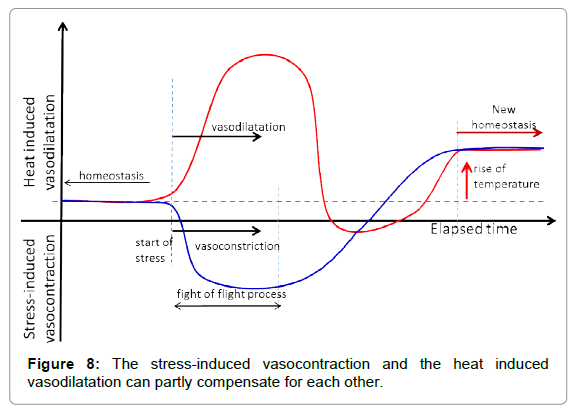
The stress-caused vasocontraction and vasodilatation, as a consequence of heating in cutis, could partly be compensated by the fight or flight reaction, more easily addressing the homeostatic control (Figure 8).

Figure 8: The stress-induced vasocontraction and the heat induced vasodilatation can partly compensate for each other.

The oncothermia dose is adjusted to support the homeostatic complex equilibrium, solving the problem deviation from the normal complex feedback regulations [78]. The controlled micro-heating [79] makes it possible to introduce the dose as the absorbed power like in standard radiotherapy [80-82].

The heating is selective in the nanoscopic range of the oncothermia process, which is ideal for gradual step-up heating without overheating the tumour mass or creating macroscopic hotspots. The oncothermia step-up heating is specialized according to the patient’s sensing. The patient senses the process, and thus guides the personalised homeostatic heating up dosing. It is more patient-friendly causing as little discomfort as possible because the patient’s homeostatic control is active. The central task is to provide the proper dose. The actual protocol for the treated patient has to be optimized to the given conditions, and needs to be curatively effective together with a high standard for safety limiting the applied dose. This concept is completely different from the conventional hyperthermia goals, because instead of trying to produce isothermal volumes (equal temperature in the tumour) it uses heterogenic heating, following the heterogeneity of the tissue itself. This far-from-equilibrium heating keeps the driving force between the heated membrane rafts and its environment, pumping the heat from these nano-clusters to the cell interior.

Oncothermia is governed in a much personalised way – the patient immediately (during the treatment and sometimes afterwards) senses and notes the toxicity. The heat pain immediately limits the oncothermia dose. When the intended dose is too much, it has to be corrected via personal signalling. On the other hand, when the protocol pre-sets too small of an energy dose, then higher energy has to be applied until the patient indicates the personalised limit. Overheating is practically impossible because the surface of the skin has the highest thermal load and the heat sensing is also there. This personalised dose regulation is the main factor of the safety and together with this for the efficacy, too.

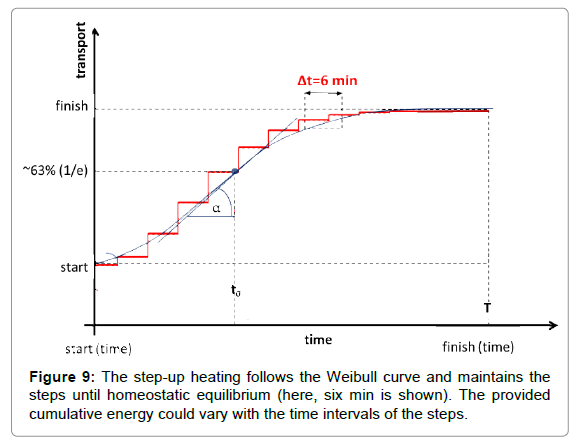
In proper step-up heating, no continuous increase of the temperature is applied. The main governing process is homeostasis, so the heating is fit to that equilibrium. A steady-state gradual heating is necessary. The physiological response time has to be considered. This characteristic time refers to when the homeostatic equilibrium is re-established in the new conditions after a definite disturbance. The average wash-out time in humans is approx. five to seven minutes. Considering the transient “break” of six min, the step-up heating is shown below (Figure 9).

Figure 9: The step-up heating follows the Weibull curve and maintains the steps until homeostatic equilibrium (here, six min is shown). The provided cumulative energy could vary with the time intervals of the steps.

The personal sensing homeostatic step-up heating solves a set of problems, but at the same time, many physiologic controls could be neglected; the overall temperature remains completely under the vasocontraction threshold despite the extreme heating of the selected malignant cells. The prescribed control is actually substituted by the personal sensing, which is regarded as the best homeostatic control for the patient.

The question naturally arises regarding the reliability of subjective sensing. According to the personal homeostasis, which is the individual set of feedback mechanisms and physiological conditions of the actual patient, personal sensing is the best available method for monitoring the heating process when all parameters of thermal homeostasis are actually involved. Personal sensing is typically used to drive many of the protocols active in today’s medical treatments. When the patient is not able to tolerate the prescribed dose, it is lowered trying to fit it to the personal tolerance level. There is no reliable personalised dosing without controlling the guidance of the personal sensing.

The conditions for using personal sensing in the heating process requires a full ability to sense heat in the treated area (not modified by analgesic application), in addition to constant personal communication contact with the patient, in addition to the ability to provide immediate intervention when indicated.

Sensing heat and pain is a complex issue dominantly connected to the nociceptors [83]. Moreover, there are specific ion channels in the cell membrane of numerous cell types in animals. Their function is to sense chemical substances and heat, mostly belonging to the transient receptor potential channels (TRP channels) family [84]. These work like “nano-thermometers” of the cells [85]. In the case of the channel for sensing heat via TRP, the rise in temperature increases the energy of the thermal movement which can tear off this closing molecule, thus opening the channel. In the case of chemical sensors, like for example the VR1 ion channel for sensing capsaicin, the closing molecule is torn off in the chemical reaction. The characteristic of these ion channels is that they are cation channels, so they are permeable to positive ions, mainly Ca²⁺. Capsaicin [86] and ethanol [87] could trigger the heat sensing TRP channels.

Note, with the decrease in pH, and thus increases in the hydrogen ion concentration, pain sensing can be triggered more easily. In other words, for example, in the case of inflammation, when the non-aerobic glucose ATP reaction is dominant, the pH decreases and so the threshold for sensing pain decreases as well [88].

In addition to the cellular sensor, the major controlling organ of the temperature forming thermal homeostatic equilibrium is the skin [89- 91]. There are systemic [92] and local [93] controlling progressions. In both, controlling the blood has a central role as heat exchange media, in addition to controlling the flow for delivering thyroid hormones and controlling the metabolic activity. The systemic control could have sympathetic nerve activity, and in cold conditions acts via shivering (activity of skeletal muscle). The vasodilatation–vasocontraction balance and the sweating and pilomotor reflexes are involved both in local and in systemic reactions. The systemic sensing is based on relative temperature between the body and the environment, while the local sensing focuses on the temperature difference between the tissues.

In observing the effect of the applied RF-frequency on heat–pain sensing, the RF-current is able to sense the heterogeneity of the tissues where it flows through. The current has two components: the ohmic and the capacitive parts. The ohmic current flows mainly in the ionic solution of the extracellular space, where the ionic displacements create the current. The capacitive current excites the dipoles, and the orientation change in them creates the capacitive current, thus this part of the current dominantly flows in the membranes. While the quantity of the complete consequent current is unchanged, the current components might vary from tissue to tissue.

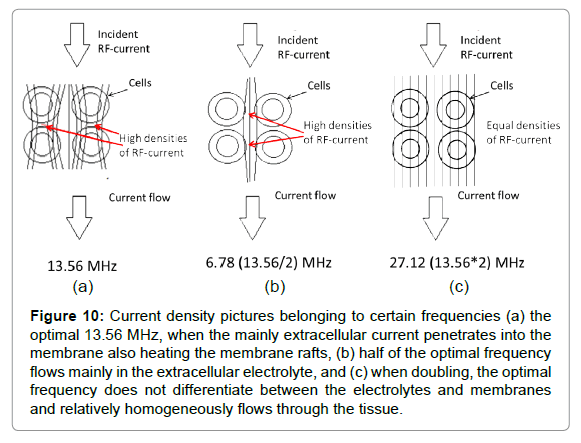
The optimal frequency is around 10 MHz [94-96], which we approach using the standard for medical use: 13.56 MHz [97]. In consequence of the complex RF current, when using half of the optimal frequency, the RF current will flow dominantly in the extracellular matrix, while in cases of doubling the optimum it will not be selective at all (Figure 10).

Figure 10: Current density pictures belonging to certain frequencies (a) the optimal 13.56 MHz, when the mainly extracellular current penetrates into the membrane also heating the membrane rafts, (b) half of the optimal frequency flows mainly in the extracellular electrolyte, and (c) when doubling, the optimal frequency does not differentiate between the electrolytes and membranes and relatively homogeneously flows through the tissue.

On the optimal 13.56 MHz frequency, the cell membrane and the heat sensing ion channels are locally heated, leading to functional sensing heat and pain. The heating is local in the cell membrane and the heat- and pain sensing is also locally connected to this.

The nanoselection of malignant cells via oncothermia allows us to return to the dosing “gold standard,” which is also applied in radiotherapy. This energy-based dose is personalised with accurate step-up heating taking into account the wash-out time and the personal sensing of the patient. The emerging new immune-oncologic connections of nanoselection [98-100], will probably change the personalization taking the immune-status of the patients into account additionally to the actual physiological parameters.