Mathematical Model for Monkeypox Virus Transmission Dynamics
Received: 04-Jul-2018 / Accepted Date: 13-Jul-2018 / Published Date: 20-Jul-2018 DOI: 10.4172/2161-1165.1000348
Keywords: Monkeypox; Equilibrium; Stability; Basic reproductive number
Introduction
Monkeypox (MPXV) is a rare viral disease that presents patients with fever and distinctive skin rash. The disease is a viral zoonosis that is transmitted to humans by animals. MPXV is transmitted in cycles that include the natural host of the virus – animals that remain largely unknown [1].
Monkeypox (MPXV) was initially noticed in State Serum in Copenhagen, Denmark during an outbreak of a pox-like disease in 1958. Since the identification of the disease, it has been discovered that MPXV has the ability to infect and breed the disease into a large number of animals within mammals in pan-geographical populations. The first recorded case of MPXV on human was recorded in 1970 on a 9-year-old boy in the Democratic Republic of Congo (DRC). The confusing trend is how a disease that was discovered in Denmark became endemic to Central and West Africa that had no prior trace of MPXV [2].
The history of the MPXV has since focused on Africa as numerous outbreaks has occurred in remote areas of the Congo Basin and West Africa where recent writings has considered the disease to be endemic. While majority of the outbreak has occurred in this region between 1996 and 1997, there seem to be periods of gap on the outbreak. This gap is also geographical due to the 2003 outbreak of MPXV in United States [3]. While this was considered to be the first outbreak outside Africa, literature has failed to make a connection of how a disease that was first discovered in Denmark is suddenly endemic to Africa. The report noted that MPXV entered into the United States when exotic African rodents were imported as pets into the United States and it then spread to household pets such as dogs, cats and other household animal that are highly susceptible to the infection. In the eighteenth and nineteenth century, the medical treaties in England, children were selected based on race and income on who receives the smallpox vaccine to keep the disease from the rich but contain it among the poor and mixed race populations [4]. So in distributing the anti-smallpox technologies, the medical community focused exclusively on the elite, white, European children, but relied on the British working class, orphaned, mixed-race, and native colonial children as subjects to study the impact of the disease without any form of vaccination. Similar to Tuskegee Airmen study which was designed to determine the natural course of untreated latent syphilis in some 400 African American men in Tuskegee, Alabama. These airmen were injected with the virus that causes syphilis. A controlled group of 200 uninfected subjects were also recruited.
In New Zealand, there are clear disparities in mortality across both ethnic and socioeconomic groups. Such close relationship between ethnicity and socioeconomic position needs to be acknowledged in understanding the Monkeypox (MPXV) patterns. The New Zealand and Tuskegee and studies have provided us with examples of unethical practice for a generation of researchers, medical reporting and indeed health agencies and ministries across the globe. The Cartwright Inquiry in New Zealand was prompted by the cervical cancer study and in the United States, the Tuskegee study gave rise to the Belmont [5]. I think it is time that world leaders seek similar report as to how diseases such as Monkeypox (MPXV) was discovered in Copenhagen, Denmark is suddenly endemic to West African and District of Angola in Africa. The jury is still out on how the monkeys in State Serum Institute in Copenhagen, Denmark, found their way to African after the 1958 discovery of the pox-like disease among local monkeys.
Since MPXV is a virus, the primary mode of transmission is generally through direct contact with an infected person through some form of body fluids such as blood, or cutaneous or mucosal lesions. So when an infected person comes in contact with a human, animal or material contaminated with the virus, the infection can gain entrance through eyes, nose, mouth, broken skin. Infections have been known to occur through the handling of infected animals such as rats, monkeys, squirrels since such rodents are the main reservoirs for MPXV. It is therefore important for individuals to be careful not to eat uncooked meat to avoid injecting an infected animal [6].
Human-to-human infection may occur as a result of close contact with the respiratory tract secretions of an infected person. Such transmission occurs primarily through contact with respiratory droplets, due to prolonged face-to-face contact while kissing or engaging in a sexual act. Contact with body fluids either through sex or with skin lesions of an infected person bodily fluid appears to be additional modes of transmission [7].
Recent literature such as the prairie dog-human monkeypox model has eluded to the fact that infected animals are capable of transmitting the MPXV through multiple channels of exposures bringing about successive infections. Consequently, two different clads, the Congo Basin and the West African clades were identified [8,9]. The Congo Basin clad is said to be more virulent, yet the study was unable to prove that the infected animals could infect potential victims exclusively through the respiratory mode of transmission.
The period of incubation for the Monkey-pox virus are 10–14 days, but on the average its 12 days. In a clinical experiment, the incubation period for infected black tailed dogs is between 4 to 13 days, in prairie dogs, it is 11 to 18 days, in infected ground squirrels, it is 4 to 5 days and in cynomolgus monkeys, the incubation period is 3 to five days. In humans, the period between infections to onset of symptoms of monkeypox usually ranges from 6 to 16 days, but can frequently range from 5 to 21 days.
The symptoms developed by some patients who may have contracted monkeypox include severe swell in their lymph nodes prior to the appearance of the rash, the distinctive feature of MPXV that set it apart from similar diseases. Monkepox symptoms are in some way similar to smallpox, but milder as it begins with a slight fever, muscle aches, exhaustion and headache [10]. The symptoms of small pox also differ in that it does not cause causes the lymph nodes to swell (lymphadenopathy) as does MPXV.
Since the incubation period for monkeypox is usually 7−14 days but can range from 5−21 days, the illness begins with swollen lymph nodes, Fever, Chills, Headache, Muscle aches, Exhaustion, and sometimes Backache. After the patients has developed fever and chills, within 3 days, the patient usually begins to develop the signature rash that MPXV is known for beginning from the face and spreading to the rest of the body. The lesions progress through the body in following stages - Macules, Papules, Vesicles, Pustules, and Scabs before falling off [11]. The disease cycles last about 2 to 4 weeks for the many that survive it. As many as 1 in 10 infected patients dies from this illness.
Much work has not been done in the area of mathematical model for monkeypox. A mathematical model for the dynamics of pox – like infection was developed. The model consists of coupled SIR model for non-human primate and human populations. The stability of the equilibria was analyzed using Lyapunov functions and center manifold theory. They simulated the effects of poor nutrition on the recovery rate [12]. It was recommended in their work that chicken pox vaccination should be re-introduced in monkeypox endemic areas. In this paper, we introduced the vaccination compartment in the human sub-population, evaluated the impact of such program. Also we assess the model for existence or otherwise bifurcation phenomena.
Materials and Method
Model formulation
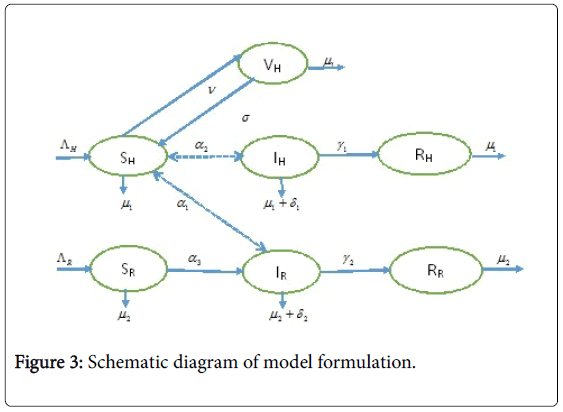
Our model consist of the human sub-population given by NH and reservoir sub- population given by NR such that NH=SH+VH+IH +RH and NR=SR+IR+RR, where SH is Susceptible Human, VH is the Vaccinated human, IH is the Infected human, RH is the Recovered human, SR is the Susceptible reservoir, IR is the Infected reservoir and RR is the Recovered reservoir. Our force of infection for the human sub-population is given by 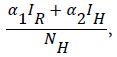 where α1 is the product of contact rate from reservoir to human c1 and the probability of an infection from reservoir to human β1, α2 is the product of the contact rate from human to human c1 and the probability of transmission from human to human β2. We assume a proportion σ of the vaccinated individual fail and returns to susceptible while (1-σ) successful vaccinated goes to the recovered human as well as a proportion ϒ1 of infected human who recover with life-long immunity (Table 1). Similarly the force of infection for the reservoir is given
where α1 is the product of contact rate from reservoir to human c1 and the probability of an infection from reservoir to human β1, α2 is the product of the contact rate from human to human c1 and the probability of transmission from human to human β2. We assume a proportion σ of the vaccinated individual fail and returns to susceptible while (1-σ) successful vaccinated goes to the recovered human as well as a proportion ϒ1 of infected human who recover with life-long immunity (Table 1). Similarly the force of infection for the reservoir is given 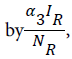 where α3 is the product of the contact rate between reservoir and reservoir c3 and the probability of transmission from reservoir to reservoir β3.
where α3 is the product of the contact rate between reservoir and reservoir c3 and the probability of transmission from reservoir to reservoir β3.
| Parameter | Meaning | Value | Reference |
|---|---|---|---|
| ∧H | Recruitment rate of human | 0.029 yr-1 | [2] |
| ∧R | Recruitment rate of mosquito | 0.2 yr-1 | [2] |
| α1 | Infection rate, from reservoir to human | 0.00025 yr-1 | [1] |
| α2 | Infection rate, from human to human | 0.00006 yr-1 | [1] |
| α3 | Infection rate, from reservoir to reservoir | 0.0027 yr-1 | [1] |
| μ1 | Natural death rate of human | 1.5 yr-1 | [3] |
| μ2 | Natural death rate of reservoir | 0.002 yr-1 | [1] |
| σ | The proportion of unsuccessful vaccinated human | 0.1-1 | Varies |
| ϒ1 | Recovery rate of infected human | 0.83-0.93 yr-1 | [3] |
| ϒ2 | Recovery rate of infected reservoir | 0.6 yr-1 | [1] |
| δ1 | Death rate of human due to infection | 0.01-0.17 yr-1 | [3] |
| δ2 | Death rate of reservoir due to infection | 0.4 yr-1 | [1] |
| Ѵ | The proportion of susceptible human that receives the vaccine | 0.1-1 | Varies |
Table 1: The parameters are shown in the given table.
The parameters, schematics diagram and the model equations are presented below (Figures 1 and 2).
The model equation
Sequel to the model formulation, parameters and the schematic diagram, Figure 3, we proposed the following equations;
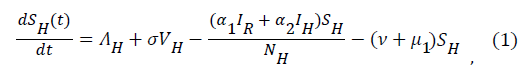
 (2)
(2)
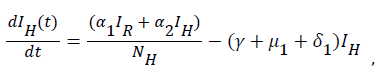 (3)
(3)
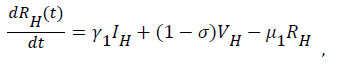 (4)
(4)
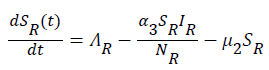 (5)
(5)
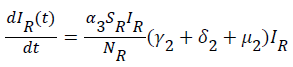 (6)
(6)
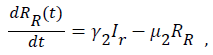 (7)
(7)
Boundedness and positivity of solutions
Consider the region;
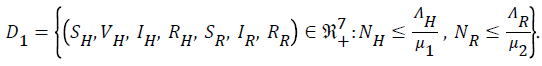
It can be shown that the set D1 is positively invariant and an attractor of all positive solutions of the system (1) to (7)
Lemma 1: The region D1 is invariant for the system (1) to (7)
Proof: The rate of change of the total human population is given by
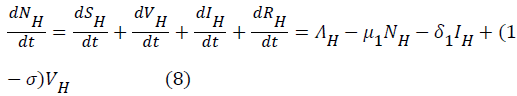
While the rate of change of the total reservoir population is given by
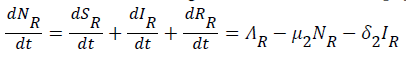 (9)
(9)
By standard comparison theorem, (Lakshmikanthan, V., Leela, S., & Martynyuk, A. A. 1989)
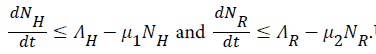 Using the integrating factor method we have
Using the integrating factor method we have 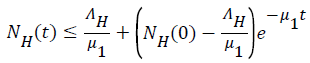 and
and 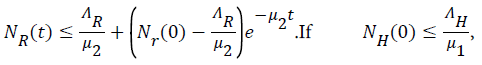 then
then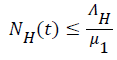 and if
and if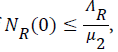 then
then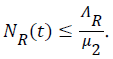
Then all solutions enter D1 in finite time and remain non-negative for non-negative initial conditions. In this region, the system (1) to (7) is said to be well posed mathematically and epidemiologically.
Positivity of solutions
Lemma 2: Let the initial data for the model (1) be SH(t)>0, VH(t)>0, IH(t)>0,RH(t)>0, then the solutions SH(t), VH(t), IH(t), RH(t), SR(t), IR(t), RR(t) of the model with positive initial data will remain positive for all time t>0.
Proof: Let t1=Sup{t>0:SH(t)>0, VH(t)>0, IH(t)>0, RH(t)>0, SR(t)>0, IR(t)>0 and RR(t)>0}>0
From system (1),
Where 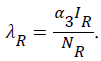 Using the integrating factor method with
Using the integrating factor method with

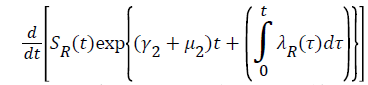

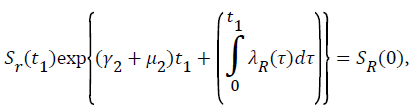
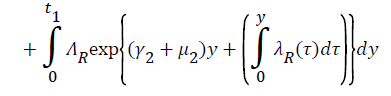
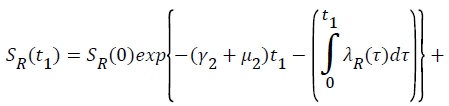
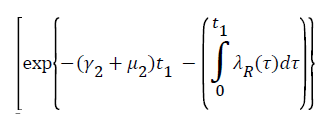
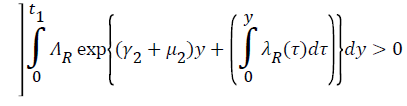
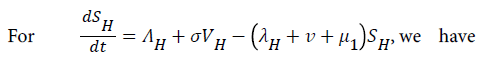
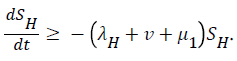
For 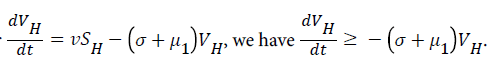
For 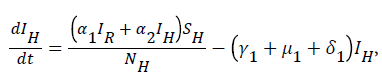 we have
we have
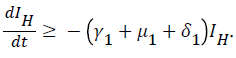
For 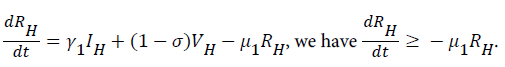
For 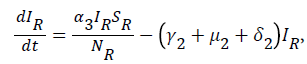 we have
we have
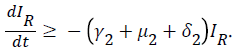
For 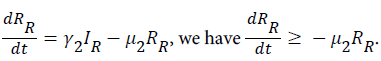
Similarly, we can show that SH(t)>0, VH(t)>0, IH(t)>0, RH(t)>0, IR(t)>0 and RR(t)>0.
Local stability of disease-free equilibrium: The disease-free equilibrium of the model is obtained by setting the right hand side of the model (10 to (7) to zero and solving the resulting equation to obtain;
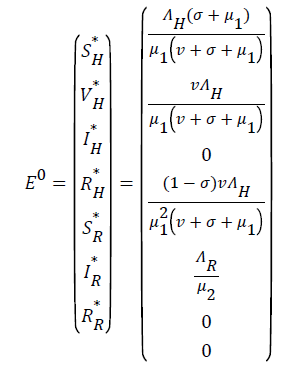 (10)
(10)
The stability of E0 is established using the next generation operator method on the system (1) to (7)
Using the next generation matrix method as described in (Driessche & Wathmough, 2002) the matrices F and for the new infection terms and the remaining transfer terms are given by
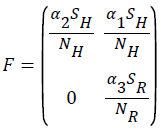
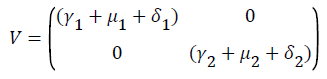
The spectral radius given by ρ(FV-1) is
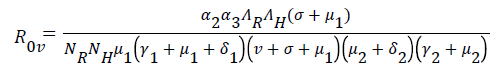 (11)
(11)
Theorem 1: The disease free equilibrium is locally asymptotically stable when and unstable when
Proof: The local stability of the disease free equilibrium is a direct consequence of (Driessche et al., 2002) and shows that the model equations (1) to (7) satisfies five assumptions in of (Driessche et al., 2002), and that ends the proof.
Global stability of disease free equilibrium: To ascertain the global stability of the disease free equilibrium (DFE) we adopt the approach [12]. Consider the infected compartment of the model equations (1) to (7).
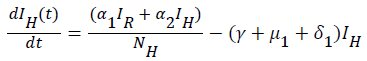 (12)
(12)
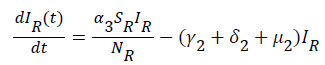 (13)
(13)
let Fi be the rate of appearance of new infections in compartment i and i-i+i be the difference between the rate of transfer of individual out of and into compartment i by all other means, we can write (12) and (13) in terms of Fi-Vi (rate of transfer in compartment i), taking the Jacobian of Fi and Vi as F and V respectively, we have:
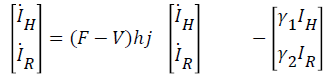
thus
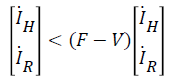 (14)
(14)
We write equations (1) to (7) as Df(xi) for i=1,2,3….,n where i=1,2,3…..,m are components in the infectious compartments, and m<n. Using E0 as the DFE which is locally asymptotically stable and considering conditions (i) to (v) in Lemma 1 in (Driessche et al., 2002) we rewrite the Jacobian matrix of (1) to (7) at E0 as a block matrix;
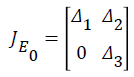
where Δ is a non - singular matrix (F – V ) and Δ3 has eigenvalues with negative real parts. Based on Driessche et al., inequality (14) is stable when R0<1. And it follows from Musekwa et al. and Nampal et al. that as t→ ∞, then (IH,IR), t→E0 which implies that E0 is globally asymptotically stable (GAS).
Sensitivity analysis
Here we present the sensitivity index of the parameters of the basic effective reproductive number (Rov). Sensitivity tells us how important each parameter is to disease transmission. Such information, is crucial not only to experimental design, but also to data assimilation and reduction of complex nonlinear model [13]. Sensitivity Analysis is commonly used to determine the robustness of model prediction to parameter values, since there are usually errors in data collection and presumed parameter values. It is used to determine parameters that have high impact on the (Rov) and should be targeted by intervention strategies. Sensitivity indexes allows us to measure the relative changes in a variable when a parameter changes. The normalized forward sensitivity index of a variable with respect to a parameter is the ratio of relative changes in the parameter when the variable is a differentiable function of the parameter. The sensitivity index may be alternatively defined using partial derivatives. The sensitivity index of our model is given below (Table 2).
| Parameter | Sensitivity index |
|---|---|
| α2 | 1.00 |
| α3 | 1.00 |
| μ1 | -0.8 |
| μ2 | 0.09 |
| σ | 0.51 |
| δ1 | 0.13 |
| δ2 | 0.99 |
| ϒ1 | 0.06 |
| ϒ2 | -0.09 |
| ∧H | 0.00 |
| ∧M | 0.00 |
| Ѵ | 0.50 |
Table 2: Sensitivity analysis index for the effective basic reproductive number.
From Table 2, the most sensitive parameters of (ROV) are the human to human infectious rate (α2) and reservoir to reservoir to reservoir infectious rate. This followed by death rate of reservoir μ2 [14]. This means that any policy or practice capable of reducing contact with infected human will go a long way in the control of monkeypox. On the other hand contact between infected reservoir and uninfected reservoir cannot controlled by human since most reservoirs are wild in nature. This implies that even with a very low basic effective reproductive number, monkeypox can hardly be eradicated.
Endemic equilibrium of the model
This is an equilibrium state where at least one of the infected compartments of the model is non-zero. To obtain the endemic equilibrium state we let;
 (16)
(16)
be the force of infection of human and reservoir respectively, further we let 1 H ** H ** H ** H ** R ** R ** R ** be an arbitrary equilibrium point and setting the right hand side of (1) to (7) to zero, solving we have;
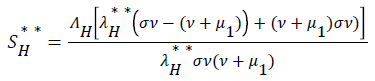 (17)
(17)
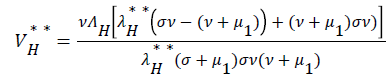 (18)
(18)
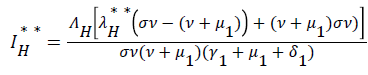 (19)
(19)
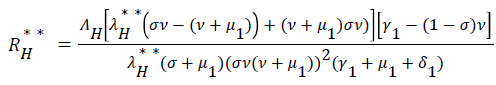 (20)
(20)
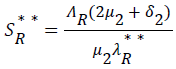 (21)
(21)
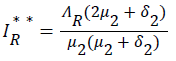 (22)
(22)
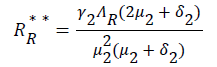 (23)
(23)
Substituting (17) to (23) into (16), we have;
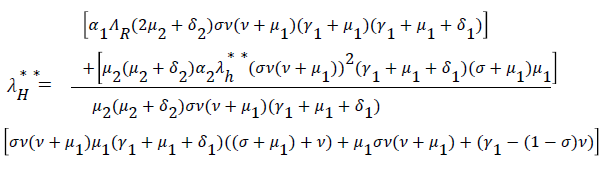
Simplifying, we have
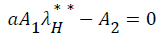 (24)
(24)
Where
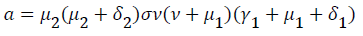
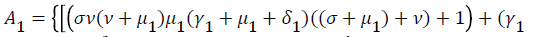
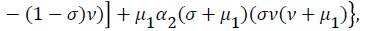
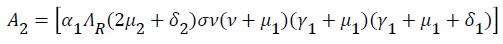
From (24) the model has one unique endemic equilibrium when ROV ≤ 1. This result rules out the possibility of backward bifurcation which requires at least two equilibria when ROV ≤ 1. The stable global stability above also precludes contemplation of backward bifurcation.
Results
Numerical simulations were conducted using the Runge-Kuta method (rkf45) for ode embedded in Matlab. The rkf45 method is a fourth-order method, meaning that the local truncation error is on the order of 0(h ), while the total accumulated error is order 0(h4). Hypothetical values were assigned to the state variables, and parameter values as given in Table 1 were used.
Discussion
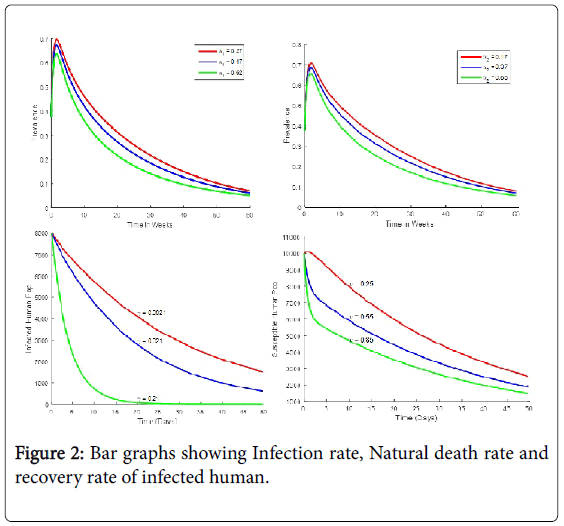
Monkeypox is a viral zoonosis infection with no specific available treatment. In the previous outbreaks, smallpox vaccine, cidofvir, ST-246 and vaccinial immune globin (VIG) were used for control. This implies that the immune system of susceptible, vaccinated or infected individuals plays a part in fighting the infection. Our simulation demonstrates the effect of weak, medium and strong immune system on the infected human [15]. The figure shows that individuals with strong immune system will recover faster than the ones with medium immune system, while on the other extreme, individuals with weak immune system recovers very slowly.
Here individual have a role to play by cultivating a good healthy living habit such as maintaining a healthy weight, exercising regularly, getting adequate sleep, minimizing alcohol intake taking balance diet etc. But communities in impoverished countries with poor access to good food, clean water, primary health care delivery system are may be handicapped [16,17]. Therefore government has a role to play here by eliminating or eradicating poverty.
Conclusion
A deterministic mathematical model was developed for the transmission dynamics of Monkeypox virus. The model incorporate imperfect vaccine compartment for the human sub-population. It was first shown that the model is mathematically and epidemiologically well posed and that the solution of the model at all time will be positive. The equilibrium of the model equation was obtained analyzed for stability. The system was shown to have one unique endemic equilibrium which is stable when ROV<1. Numerical simulation was carried it to underscore the role weak, medium and strong immune system on some epidemiological states, as well as the effect of infection and vaccination rates on the prevalence and susceptible respectively.
References
- Bhunu CP, Mushayabasa S (2011) Modelling the Transmission Dynamics of Pox-like Infections. IAENG IJAM, 41: 2.
- Bhunu CP, Garira W, Magombedze G (2009) Mathematical analysis of a two strain HIV/AIDS model with antiretroviral treatment. Acta Biotheor 57: 361-381.
- Brewer N, Borman B, Sarfati D, Jeffreys M, Fleming ST, et al. (2011) Does comorbidity explain the ethnic inequalities in cervical cancer survival in New Zealand? A retrospective cohort study. BMC Cancer 11: 132-138.
- Centers for Disease Control and Prevention [CDC] (2018) Considerations for selection and prioritization of animal specimens for laboratory testing. U.S. Department of Health & Human Services.
- Driessche VP, Wathmough J (2002) Reproductive Number and Sub-Threshold Endemic Eqilibria for Compartment Modelling of Disease Transmission. Math Biosci 180: 29-48.
- Egan C, Kelly CD, Rush-Wilson K, Davis SW, Samsonoff WA, et al. (2004) Laboratory-Confirmed Transmission of Vaccinia Virus Infection through Sexual Contact with a Military Vaccinee. J Clin Microbiol 42: 5409–5411.
- Essbauer S, Pfeffer M, Meyer H (2009) Zoonotic poxviruses. Vet Microbiol 140: 229-236.
- Falendysz EA, Lopera JG, Doty JB, Nakazawa Y, Crill C, et al. (2017) Characterization of monkeypox virus infection in African rope squirrels (Funisciurus sp.). PLoS Negl Trop Dis 11: 1-23.
- Georges A, Matton T, Courbot-Georges M (2004) Monkey-pox, a model of emergent then reemergent disease. Medecine & Maladies Infectieuses 34: 12-19.
- Hutson CL, Gallardo-Romero N, Carroll DS, Clemmons C, Salzer JS, et al. (2013) Transmissibility of the Monkeypox Virus Clades via Respirator Transmission: Investigation Using the Prairie Dog-Monkeypox Virus Challenge System. Plos ONE 8: 1-12.
- Lakshmikantham V, Leela S, Martynyuk AA (1999) Stability Analysis of Non-linear Sysytems. New York and Basel: Marcel Dekker, Inc.
- Murdoch L (2015) Carrying the Pox: The Use of Children and Ideals of Childhood in Early British and Imperial Campaigns Against Smallpox. Journal of Social History 48: 511-535.
- Â Musekwa S, Nyabadza C, Chiyaka C, Das P, Tripathi A, et al. (2011) Modelling and analysis of the effctes of malnutrition in the spread of cholera. Mathematical and computer modelling 53: 1583-1595.
- Â Nampal H, Luboobi LS, Mugisha JTY, Obua C (2013) Mathematical modelling of liver enzyme elevation in HIV mono-infection. Math Biosci 242: 77-85.
- Parker S, Buller RM (2013) A review of experimental and natural infections of animals with monkeypox virus between 1958 and 2012. Future Virology 8: 129-157.
- Paul C, Brookes B (2015) The Rationalization of Unethical Research: Revisionist Accounts of the Tuskegee Syphilis Study and the New Zealand "Unfortunate Experiment". Am J Public Health 105: e12-e19.
- Powell DR, Faie J, Leclaire RJ, Moore LM, Thompson D (2005) Sensitivity Analysis of an Infectious Disease Model. International System Dynamic Conference,Boston, MA.
Citation: Emeka PC, Ounorah MO, Eguda FY, Babangida BG (2018) Mathematical Model for Monkeypox Virus Transmission Dynamics. Epidemiology (Sunnyvale) 8: 348 DOI: 10.4172/2161-1165.1000348
Copyright: © 2018 Emeka PC, et al. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.
Share This Article
Recommended Journals
Open Access Journals
Article Tools
Article Usage
- Total views: 6577
- [From(publication date): 0-2018 - Mar 31, 2025]
- Breakdown by view type
- HTML page views: 5266
- PDF downloads: 1311