Common Mating Design for Hybrid Development
Received: 01-Jan-2022 / Manuscript No. acst-22-51147 / Editor assigned: 04-Jan-2022 / PreQC No. acst-22-51147 (PQ) / Reviewed: 18-Jan-2022 / QC No. acst-22-51147 / Revised: 24-Jan-2022 / Manuscript No. acst-22-51147 (R) / Accepted Date: 21-Jan-2022 / Published Date: 31-Jan-2022 DOI: 10.4172/ 2329-8863.1000491
Abstract
The most important design used in plant breeding to produce progeny is the mating design. Plant breeders and geneticists use a number of mating techniques to achieve their goals. The choice of a proper mating design is crucial for plant breeding success. Plant breeders experimented with various mating designs and arrangements in order to produce progenies. With the major aims of gathering genetic information and producing plant cultivars to obtain the base population, mating designs play a critical role. The choice of mating pattern and genetic resources are crucial in conventional crop enhancement programs. Several factors influence mating design selection, including time, space, study aims, and other relevant issues. The analysis of variance gives a quick and easy way to understand the many types of mating designs. In general, combining ability is important in estimating genetic components and selecting appropriate parents and hybrids to increase crop output. The capacity to combine traits is crucial in determining the breeding strategy to develop desired qualities There is a chance to improve parents through selection when the general combining ability to specific combining ability is greater than unity, and when the general combining ability to specific combining ability is less than unity, the breeding procedure is designed to improve desirable traits through heterosis breeding. To develop agricultural development programs, gene activities are examined and studied utilizing various mating patterns as genetic expressions. To improve the required desirable features, hybridization with the use of mating is very important to widen the genetic bases of the population.
Keywords
Mating Design; Hybrids; Dominance; Additive Gene; Combining Abili
Introduction
Mating design is a procedure for developing progenies and determining the type gene actions involved in trait inheritance. Breeders and geneticists have experimented with various mating patterns in order to create superior varieties with desirable characteristics [1]. During hybrid development, mating design is used to determine the distinct types and magnitude of gene effects (additive, dominance, and epistatic). Mating designs are used to determine the types of gene actions involved in the inheritance of the traits in question, as well as the combining abilities of the parental populations involved in cross-breeding. In order to address the growing need for enhanced technologies, mating designs are now playing an important part in the development of the highest performing and superior genotypes in various arrangements and cross combinations by altering the genetics of crops [2].
Initially, mating designs were used to assess genetic variance components such as additive, dominance, and epistatic variances. For the generation of ideal genotypes to be effective, appropriate parents must be chosen and a mating design must be used. However, mating design selection is influenced by the type of crossing used, the type of pollination used, the type of pollen dispersion used, the presence of a male sterility mechanism, and the target population size. Mating design is used to assess genetic diversity based on the investigation’s objectives, space, time, and biological limiting constraints [3]. Mating design is primarily concerned with providing information on genetic variances, producing a genetic population as a foundation for improvement, identifying prospective genotypes, and calculating genetic gain [4]. To address any plant breeding-related research concerns, such as whether genetic diversity is significant, any specific mating design is used. How much of the variation is due to hereditary factors and how much is due to environmental factors? What type(s) of gene(s) have an impact on significance?
Mating design allows for the development of a high number of crosses in order to broaden the population’s genetic base and increase efficiency and effectiveness. The most commonly utilized genetic variance analyses are genetic advancements, combining ability, heterosis, heritability, correlation and regression analyses, generation mean analysis, stability analysis, and gene action [5]. The interpretation of the data in a plant breeding experiment should reflect the mating design in order to meet the specified objectives [6]. Plant breeders and geneticists use various mating designs in various configurations to develop superior improved agricultural plants as their primary and ultimate goal. Breeders can now pick genotypes with the best performance based on the performance of the progenies produced.
Combining ability is an important factor to consider when selecting appropriate inbred lines, and it is controlled by the gene action that determines the traits. General combining ability, which is the result of additive gene action effects, is used to determine a line’s average performance in hybrid formation. The general combining ability of a line is determined by the mating design, but it is essentially the difference between the mean of its progeny and the mean of all lines in the experiment [7, 8]. Non-additive gene activation causes specific combining ability, which is defined as the ability of crosses to perform better or worse than expected based on the average performance of the lines involved. Estimates of combining ability and gene activity are crucial for a successful plant breeding effort [9, 10].
Different genetic components of variation are examined using mating design to estimate quantitative characters. Appropriate mating design should be employed to develop the best progenies for the success of improvement [11]. Plant breeders are especially interested in improving crops through hybridization, and choosing better parents is crucial for yield and other desirable features. The review’s goal was to better understand common mating designs so that superior genetic materials could be identified and breeding programs could be designed for future enhancement.
Mating Design
Plant breeding for agriculture and bio-science uses a schematic cross between two groups or strains of plants to produce progenies. Plant breeders must examine additive and non-additive components of genetic variance in order to devise successful selection procedures for improving quantitative traits. The mating design for quantifying genetic variants should consider the study’s objectives, as well as time, space, cost, and other biological limitations. Plant breeders may utilize a variety of mating tactics to identify genetic features in populations. To create superior plants, plant breeders and geneticists use a variety of mating techniques and arrangements [12].
Plant breeders are concerned about selecting better parents for yield and other desirable features that will combine effectively when crossed for developing crops through hybridization. Mating designs are used to determine the types of gene actions involved in the inheritance of the traits in consideration, as well as the combining abilities of the parental populations involved in cross-breeding [13]. As a result, in addition to providing information about the nature of gene action, combining ability investigations also allows for the classification of selected parental material in terms of breeding behavior. With advancements in biometrical genetics, numerous strategies for estimating combining ability have been proposed.
Major Mating Designs in Plant Breeding and Genetics
Mating design refers to the process of developing offspring in plant breeding. Plant breeders and geneticists use a variety of mating patterns and combinations, both theoretically and practically, for a number of purposes. Biparental progenies (BIP), poly-cross, Topcross, North Carolina (I, III, III), Diallel (I, II, III, and IV), and Line x tester design are the six types of mating designs described thus far [14,15]. Individuals are randomly selected and crossed in all mating schemes to produce half-sibling or full-sibling progenies.
Bi-Parental Mating
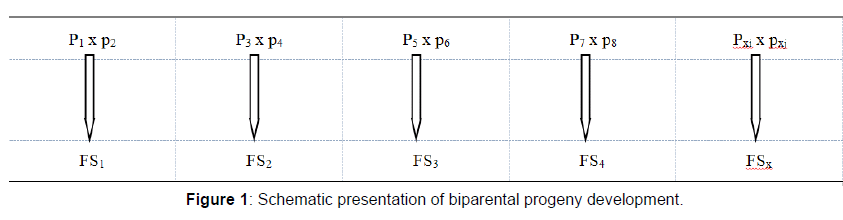
The bi-parental design, also known as paired crossing design, is the most basic mating design [16]. In this pattern, the breeder randomly selects a large number of plants (n) and crosses them in pairs to produce 1/2n full-sib families (Acquaah, 2012). Bi-parental mating is also known as paired crossing design. The mating design gives information needed to assess whether variation within a population is significant for a long-term selection program (e.g., cross-pollinated species) with minimal effort and cost, as well as information needed to create variability (e.g., cross-pollinated species) [17].
On the other hand, the design is unable to provide information on the type of genetic variation. Individuals from a random mating population are selected at random and mated in a bi-parental mating arrangement. Individual plants can usually be crossed reciprocally to produce progenies that can be bulked for testing in various environments. Several crosses are necessary to obtain precise measurements and adequate interpretations in relation to the reference population. If n parents are used, the total number of crossings equals n/2 [18] (Figure 1).
In the formulation of an effective breeding program for genetic improvement, bi-parental mating helps in the creation of variability and establishes the relative importance of genetic components of variance (additive and dominance components of variance) as well as the expected response to trait selection. It’s the simplest setup, with a random number of P plants grouped together to form 12P families. Only once are the parents mated in pairs. As a result of their pregnancy, P parents had 12 full-sibling families [19]. When evaluating r plants per progenies families, the variance within (w) and between (b) families can be statistically analyzed (Table 1).
| Source of variation | Df | MS | EMS | ||
|---|---|---|---|---|---|
| Between families | (a/b n-1) |
|
|
||
| Within families | a/b n(r-1) |
|
σ2w | ||
| Total | a/b nr-1 | - | - | ||
| Where n and r are the number of parents and plants sampled within each cross, respectively; 2b is the covariance of full-sibs (2b=Cov FS=12VA + 14 VD + VEC) = 1r (MS1-MS2); and 2w = [2G - Cov FS] + 2EW =12VA +34 VD + VEW = MS2; is the environmental source of variation for variance within the crosses. 2b = 12VA and 2w = 12VA + VEW are the values when dominance effects are assumed to be zero. | |||||
Table 1: Analysis of variance for biparental mating design.
Poly-cross Design
A polycross is a mating arrangement that uses natural hybridization in an isolated crossing block to inter-pollinate a set of cultivars or clones [20]. Offspring from a line that was outcrosses with other selected lines in the same nursery are referred to as “polycross.” This plan is for organically crossing a group of cultivars in a small area. If an isolation block is not available, hand-crossing is required, and the entrance must be planted to allow for the requisite inter-pollination. The mating design is frequently employed to create synthetic cultivars and can also be utilized in recurrent selection processes to recombine selected entries or families. Self-pollination is avoided because the design allows each clone or parent in the block an equal chance to organically cross with one other [21]. However, a good design of the polycross block is required to achieve this goal. It gives each entry an equal chance of being crossed with every other entry. It is vital that the entries in the crossing block are evenly distributed and ordered randomly [15, 20].
The ability to develop synthetic cultivars, recombine selected genotypes in a recurring selection phase, and test the parent genotypes’ general combing ability are all advantages of the polycross design [22]. The general combining abilities assessed are mostly for maternal parents, and differences found in a progeny can be partitioned into within and between maternal parents, assisting in heritability estimation. The variance components and, as a result, the general combining ability are computed using the mean performance of the progenies of any female parent in the polycross (GCA) (Table 2).
| Sources | Df | MS | Expected mean square | Variance components |
|---|---|---|---|---|
| Progenies | g-1 | M1 | σ2e + r σ2prog | σ2prog = Cov (HS) = 1+F/4 σ2A |
| Blocks | r-1 | M2 | - | - |
| Error | (g-1)( r-1) | M3 | σ2e | σ2e = σ2 |
| When the parents are non-inbred, F= zero, and the variance component 2 prog is an estimate of (1+F)/4 2A. When analyzing a large number of genotypes in cross-pollinated species, Polycross design is useful. After that, the selection is based on half-sib progeny means. | ||||
Table 2: ANOVA table of Polycross design with many replications.
Top Cross Design
A top cross is a cross between a variety, inbred line, or single cross and a common pollen parent, such as a variety, line, or clone. The chosen plants are crossed with a common tester (or testers) with a track record in open pollination. Both narrow-based and broadbased tests are acceptable; the tester’s parent must have a well-known genetic history. The top cross mating method involves mating a group of selections, lines, or clones to a common parent (tester), which can be a cultivar, an inbred line, a single cross, or something else entirely, and the tester is the same for each mating.
Because all crosses employ the same tester, all offspring families are half-sibs; hence, top cross mating design allows for GCA evaluation for the group of lines, clones, or selections participating in the crosses. The top cross mating pattern is most widely utilized in cross-pollinated crops like maize, and it is frequently an inbred-cultivar cross. The design is often used to perform preliminary assessments of the breeding potential of new maize accessions. Top cross has long been used to assess the combining skills of new inbred lines. When there are n inbreds, the number of feasible crosses is n x 1. Only GCA information is provided by top cross progenies, not SCA. It’s a quick and easy way to test how well inbred lines work together before using them in single-cross yield studies. This design is likely the simplest sort of mating design that can enable preliminary rapid screening of genetic stocks since it contains the least crossing load and basic statistical analysis (Table 3).
| Source of variation | Df | Mean Squares | Expected Mean Squares | Variance of relatives |
|---|---|---|---|---|
| Progenies | g -1 | M1 | σ 2 e + rσ2prog | σ2 prog = CovHS = [(1+F)/4] σ2A |
| Blocks | r -1 | M2 | - | - |
| Error | g – 1)(r – 1) | Me | σ2e | σ2e = σ2 |
| When the parents are non-inbred, F= zero, the variance component 2prog is an estimate of (1+F)/4 2A computed from 2prog = V (m1) + V (m2). | ||||
Table 3: Analysis of variance for top cross progenies.
North Carolina Design
One of the most useful mating designs for measuring genetic diversity and crop selection is the North Carolina design. It’s ideal for self-pollinated crops with a lot of flowers since the mating pattern creates a large number of progenies. NC Design I, NC Design II, and NC Design III are the three separate mating systems used in North
Carolina design.
North Carolina Design I: NC Design I am only suitable for evaluating genetic variance in a linkage-equilibrium randomly mated population. It’s a flexible design that can be used for theoretical as well as practical plant breeding. It’s frequently used to evaluate fulland half-sib recurrent selection, as well as to compute additive and dominant variances. It’s not possible to breed species that can’t produce significant amounts of seed since repetitive evaluation experiments require enough seed. It applies to self-pollinated and cross-pollinated species that meet the criteria. NC Design I am a hierarchical design with common and non-common parents nestled within each other. The NCI provides three statistics in comparison to biparental and polycross designs, whereas the polycross and biparental designs only provide two (Table 4).
| Source | Df | MS | Expected MS |
|---|---|---|---|
| Sets | (s-1) | ||
| Replications in sets | s(r-1) | ||
| Males in sets | s(m-1) | M1 | σ 2e + kσ2p +rkσ2f + rkfσ2m |
| Females in males in sets | sm(f-1) | M2 | σ2e + kσ2p + rkσ2f |
| Reps x Females | s(mf-1)(r-1) | M3 | σ2e + kσ2p |
| Residual | smfr(k-1) | M4 | σ2e |
| Total | smfrk-1 |
Table 4: Format of the ANOVA table for North Carolina design I.
North Carolina Design II: Each member of one group of male parents is paired with each member of another group of female parents in this method. It’s used to see how effectively inbred lineages can blend together. The design is best suited to plants with many blossoms because one plant can be used as both male and female multiple times. In this concept, blocking is utilized to preserve all mating between a single group of men and a single group of females as a single unit. The experiment is a straightforward two-way ANOVA, with the variance split between males (m) and females (f), as well as their interaction.
This design also allows the breeder to assess both GCA and SCA. The NCII, on the other hand, does not offer epitasis or G x E interaction testing. Every progeny family in North Carolina II has halfsibling ties through both common male and common female. This is achieved using a systematic crossover technique in which n1 males and n2 females are mated in every feasible combination to generate n1n2 progeny families. Unless n1=n2, it is consequently a rectangular mating design. Reciprocal crosses can be used to investigate the consequences of motherhood (Table 5).
| Source | Df | Expected MS |
|---|---|---|
| Sets | s-1 | |
| Replications in sets | S(r-1) | |
| Between males | S(m-1) | σ2W + rσ2m x f +rfσ2m |
| Between females | S(f-1) | σ2W + rσ2m x f +rmσ2f |
| Males x females | s(m-1) (f-1) | σ2W + rσ2 m x f |
| Plots within replications | S(mf-1)(r-1) | σ2W |
| Total | Srmf-1 |
Table 5: Format of the ANOVA table for North Carolina Design II.
North Carolina Design III: Backcrossing a random sample of F2 plants to the two inbred lines from whence the F2 was descended is used in this design. It is regarded to be the most powerful of the three NC designs. Upgrades by Kearsey and Jinks, which included introducing a third tester in addition to the two inbreds, made it more powerful. F2 is evaluated using the two parental lines that serve as testers. Because F2 segregates at all loci for which the testers differ but not for any other loci, the parents, as progenitors of the F2, are exceptional testers. The F2 population is used as a reference population in NCIII mating.
The triple test cross is a variant that can test non-allelic (epistatic) interactions as well as estimate additive and dominance variance, something that the other designs couldn’t do. The first portion of the study is an epistasis test, while the second half examines the significance and calculates the additive and dominant components of variance. Because the NCIII is a particular variant of the NCII, the ANOVA is similar, with one exception: the two testers are not a random sample from any population, but rather two extremely unique lines that are the progenitor of the F2 (Table 6).
| Source | Df | MS | Ems |
|---|---|---|---|
| Replications | (r-1) | ||
| Parents/Testers (T) | 1 | MST | σ2W + rσ2Tm + mrk2T |
| F2 (m) | m-1 | MSm | σ2W + 2rσ2m |
| T x M | m-1 | MSTm | σ2W + rσ2Tm |
| Within FS families | (r-1)(2m-1) | MSW | σ2W |
| Total | 2mr-1 |
Table 6: Format of the ANOVA table for the North Carolina design III.
Diallel design
A complete diallel mating design allows the parents to be crossed in every possible combination, including selfs and reciprocals. This is the mating pattern required for achieving Hardy–Weinberg equilibrium in a population. The diallel is the most extensively used and utilized mating design for acquiring genetic data. The fact that there are two types of diallel analysis models, random and fixed, may explain why it is so popular. Parents in a random model are members of a random mating population. For examining GCA and SCA variations, a random approach works well.
When parents are fixed effects, the goal is to quantify the GCA effect for each parent as well as the SCA effect for each pair of parents. A complete diallel mating design allows the parents to be crossed in every possible combination, including selfs and reciprocals. This is the mating pattern required for achieving Hardy-Weinberg equilibrium in a population. Griffing’s diallel approaches are the most extensively utilized in diallel analysis. established four diallel methods in plants: 1) The complete diallel: parents, F1, and reciprocals Half diallel: parents and F1’s, full diallel: F1’s and reciprocals, full diallel: F1’s and reciprocals, full diallel: F1’s and reciprocals, full diallel: F1’s and reciprocals, full diallel: F1’s and reciprocals, full diallel: F1’s and reciprocals, full diallel: F1’s and reciprocals, full dial. F1’s each method yields a different number of progenies; the number of progeny families (pf) for procedures 1 through 4 is pf = n2, pf = 1/2n (n + 1), pf = n (n-1), and pf = 1/2n (n-1), respectively (Acquaah, 2012). These four approaches have been widely used to look at the inheritance patterns of various traits in a wide range of crops.
This mating design includes GCA and SCA information. Methods 3 and 4 employ a fixed model to produce unbiased estimates of combining abilities and gene action. When there are no genotypic reciprocal effects, this technique works well. Poor experimental design causes the majority of diallel cross issues, making data processing difficult. A higher GCA/SCA variance ratio indicates the importance of additive genetic influences, whereas a lower ratio indicates dominance and/or epistatic gene effects. Only when the aggregate analysis reveals significant GCA and SCA mean squares are individual GCA and SCA impacts assessed. In genetics and breeding, there are four diallel mating designs that are widely utilized.
Method I or full diallel design: Parents, one set of F1’s, and reciprocal F1’s made up technique I, or entire diallel design. The technique generates a total of n2 genotypes (Table 7).
| Expected mean squares | ||||||
|---|---|---|---|---|---|---|
| Source | Df | SS | MS | Model I | Model II | |
| GCA | p-1 | Sg | Mg | σ2 +2p( 1/p-1 )Σgi2 | σ2 +2( p-1/p ) σ2g+2p σ2g | |
| SCA | p(p-1)/2 | Ss | Ms | σ2 + 2/p(p-1)ΣΣsij2 | σ2 +2( p2-p+1/p2 ) σ2s | |
| Reciprocal eff. | p(p-1)/2 | Sr | Mr | σ2 +2( 2/p(p-1))ΣΣrij2 | σ2 + 2 σ2r | |
| Error | M | Se | Me | σ2 | ||
Table 7: Skeleton of ANOVA for method I diallel design.
Method II or half diallel design: This technique includes parents as well as one set of F1s that are not reciprocals. p (p+1)/2 genotypes result from this design(Table 8).
| Expected mean squares | ||||||
|---|---|---|---|---|---|---|
| Source | Df | SS | MS | Model I | Model II | |
| GCA | p-1 | Sg | Mg | σ2 +(2+p)( 1/p-1 )Σgi2 | σ2 + σ2s +(p+2) σ2g | |
| SCA | p(p-1)/2 | Ss | Ms | σ2 + 2/p(p-1)ΣΣsij2 | σ2 + σ2s | |
| Error | M | Se | Me | σ2 | ||
Table 8: Analysis of variance for method II.
Method III: One set of F1s and the reciprocals are provided in this strategy. A = p (p-1) distinct number of genotypes result from this mating scheme. It also provides fixed and random effect models for techniques I and II (Table 9).
| Expected mean squares | ||||||
|---|---|---|---|---|---|---|
| Source | Df | SS | MS | Model I | Model II | |
| GCA | p-1 | Sg | Mg | σ2 +2p(p-2)( 1/p-1 )Σgi2 | σ2 + 2 σ2s +2(p-2) σ2g | |
| SCA | p(p-3)/2 | Ss | Ms | σ2 + 2/p(p-3)ΣΣsij2 | σ2 + 2 σ2s | |
| Reciprocal eff. | p(p-1)/2 | Sr | Mr | σ2 +2( 2/p(p-1))ΣΣrij2 | σ2 + 2 σ2r | |
| Error | M | Se | Me | σ2 | σ2 | |
Table 9: Skeleton of ANOVA of Diallel method III.
Method IV: Only one set of F1s is used in this procedure. The diallel crossing system is the most prevalent. There are a total of a = p (p-1)/2 genotypes that have been tested. There are two models for different diallel techniques (Table 10).
| Expected mean squares | ||||||
|---|---|---|---|---|---|---|
| Source | Df | SS | MS | Model I | Model II | |
| GCA | p-1 | Sg | Mg | σ2 +(p-2)( 1/p-1 )Σgi2 | σ2 + 2 σ2s +(p-2) σ2g | |
| SCA | p(p-3)/2 | Ss | Ms | σ2 + 2/p(p-3)ΣΣsij2 | σ2 + 2 σ2s | |
| Error | M | Se | Me | σ2 | σ2 | |
Table 10: Skeleton of ANOVA for Diallel method IV.
Line × Tester Design
The L x T mating design is essentially a variation on the top cross design, with the exception that instead of one tester, the L x T mating design employs a large number of testers. In this concept, lines (f) and wide-based testers (m) are hybridized one-to-one, yielding f x m = fm hybrids. It is the most fundamental mating pattern, producing both full-sibs and half-sibs at the same time, in contrast to top-cross, which only produces half-sibs. Because the genotypes of liner and tester are different, it delivers SCA for each cross as well as GCA for both lines and testers [23].
It’s also used to estimate a range of gene activities that influence the expression of quantitative traits. One of the most effective approaches for predicting parents’ general combining ability (GCA) and identifying parents and crosses with high special combining ability (SCA) is to use line tester analysis. For establishing the nature and amount of gene action influencing quantitative traits, the line x tester mating design is beneficial. A line tester can be used to see if female and male lines have the potential to produce desirable hybrid combinations. It helps with the selection of parents for cultivar development or hybridization projects by providing information on the role of genes in determining desirable trait inheritance. It’s the most effective method for estimating the value of germplasm and selecting the finest parents for creating superior hybrids [24] (Table 11).
| Expected mean squares | ||||
|---|---|---|---|---|
| Source | Df | MS | Model I | Model II |
| Replication | r-1 | |||
| Lines | m-1 | M1 | σ2 +rf 1/m-1 +Σgi2 | σ2 + Vsca + rfgca(m) |
| Testers | f-1 | M2 | σ2 +rm 1/f-1 +Σgj2 | σ2 + rVsca + rmgca(m) |
| Line X Tester | (m-1)(f-1) | M3 | σ2 +r[1/(m-1)(f-1)]+ΣΣsij | σ2 + rVsca |
| Error | (r-1)(mf-1) | M4 | σ2 | σ2 |
Table 11: Skeleton of ANOVA for Line x Tester Design.
Conclusion
The most fundamental and pre-requisite stage in a plant breeding program is to create genetic variety in order to improve agricultural plants through hybridization. Inter-specific hybridization, mutation, polyploidy, and recombination all contribute to genetic variety. Hybridization is the most important of these for increasing genetic variety and ensuring the success of a crop development effort. Plant breeding techniques require the selection of optimal mating design and parents in order to yield viable genetic materials. Hybridization from superior parents is used to create crosses with desirable features like yield and other associated traits.
The capacity to combine parents during the hybridization process in order to pass desired genes to the next generation is known as combining ability. Variation due to general combining ability and variance due to specific combining ability are the two major categories of combining ability. Mating design is extremely important for estimating combining ability and determining gene activities involved in trait inheritance. Breeders and geneticists use a variety of mating designs and arrangements to generate superior crop plants in plant breeding programs.
To design appropriate selection strategies to increase quantitative traits, both additive and non-additive genetic components are determined and quantified. Various mating designs might be applied in order to produce the best and superior progenies. In general, mating designs are created to estimate and determine the type and quantity of genetic components in order to devise the best breeding processes for enhancing the crop plant. As a result, selecting a mating design is crucial for plant breeding projects to progress and succeed in the future. General and specific combining ability variances can be defined in terms of population genetic variance, which can be further divided into additive and non-additive components of variation.
References
- Acquaah G (2012) Principles of plant genetics and breeding. Wiley-Blackwell, Oxford.
- Aly RSH (2013) Relationship between combining ability of grain yield and yield components for some newly yellow maize inbred lines via line x tester analysis. Alex J Agric Res 58: 115-124.
- Comstock RE, Robinson HF (1952) Estimation of average dominance of genes. Heterosis 2:494-516.
- Griffing B (1956) Concept of general and specific combining ability in relation to diallel crossing systems. Aust J Biol Sci 9: 463-493.
- Hallauer AR, Miranda Filho JB, Carena MJ (2010) Hereditary variance: mating designs. In quanti gen in maize bre: 81-167.
- Hill WG (2010) Understanding and using quantitative genetic variation, Philosophical Transactions of the Royal Society B. Biolog Sci 365: 73-85.
- Johnson GR, King JN (1998) Analysis of half diallel mating designs me: a practical analysis procedure for ANOVA approximation. Silvae Genetica 47: 74-79.
- Kearsey MJ, Pooni HS (1996) The genetical analysis of quantitative traits. Recent res sci technol 9: 34-56
- Khan SA, Ahmad A, Khan A, Saeed M (2009) Using line x tester analysis for earliness and plant height traits in sunflower (Helianthus annuus L.). Recent res sci technol 1: 202–206.
- Clerg EL (1966) Significance of experimental design in plant breeding. Significance of experimental design in plant breeding. Biolog Sci 365: 72-86
- Mahalingam A, Robin S, Mohanasundaram K, Pushpam R (2011) Studies on genetic architecture of biparental progenies for important yield attributes in rice (Oryza sativa L.). J Plant Breed Crop Sci 3: 296-301.
- Mather K, Jinks JL (1982) Components of variation: in biometrical genetics. J Plant Breed Crop Sci 2:135-175.
- Mindaye TT (2016) Genetic differentiation, heterotic performance and grain yield determinate traits of locally adapted sorghum genotypes in contrasting environments in Ethiopia. Recent res sci technol 10: 34-56
- Mosa HE (2010) Estimation of combining ability of maize inbred lines using top cross mating design. J Agric Res 36: 1-14.
- Mumtaz A, Sadaqat HA, Mubarik MK, Saif-ul-Malook MAN (2015) A review on genetics of seed yield and quality related traits in Brassica group. Biolog Sci 2:1-6.
- Nduwumuremyi A, Tongoona P, Habimana S (2013) Mating designs: helpful tool for quantitative plant breeding analysis. Int J plants breed gen 1:117-129.
- Panhwar SA, Baloch MJ, Jatoi WA, Veesar NF, Majeedano MS (2008) Combining ability estimates from line x tester mating design in upland cotton. Proc Pakistan acad sci 45: 69-74.
- Rashid M, Cheema AA, Ashra M (2007) Line x tester analysis in Basmati rice. Pak J Bot 39: 2035-2042.
- Saif-ul-malook MA, Ali Q, Mumtaz A (2014) Inheritance of yield related traits in maize (Zea mays) under normal and drought conditions. Nat Sci 12: 36-49.
- Saladaga FA (1989) The theoretical basis and practice of polycross as used in sweet potato. Res Develop for Small Far: 83-98.
- Shattuck VI, B Christie, C Corso (1993) A principle of Griffing’s combining ability analysis. Genetica 90: 73- 79.
- Singh RJ, Kollipara KP, Hymowitz T (1993) Backcross (BC2‐BC4). Derived Fertile Plants from Glycine max and G. tomentella Intersubgeneric Hybrids. Crop Sci 33: 1002-1007.
- Sleper DA, Poehlman JM (2006) Breeding field crops.
- Stuber CW (2004) Breeding: mating designs. Encyclo plant cro Sci 1:225-228.
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Google Scholar, Crossref, Indexed at
Citation: Begna T, Yali W (2022) Common Mating Design for Hybrid Development. Adv Crop Sci Tech 10: 491. DOI: 10.4172/ 2329-8863.1000491
Copyright: © 2022 Begna T, et al. This is an open-access article distributed under the terms of the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are credited.
Select your language of interest to view the total content in your interested language
Share This Article
Recommended Journals
Open Access Journals
Article Tools
Article Usage
- Total views: 6408
- [From(publication date): 0-2022 - Oct 16, 2025]
- Breakdown by view type
- HTML page views: 5612
- PDF downloads: 796