ISSN: 2165-7025
Journal of Novel Physiotherapies
Make the best use of Scientific Research and information from our 700+ peer reviewed, Open Access Journals that operates with the help of 50,000+ Editorial Board Members and esteemed reviewers and 1000+ Scientific associations in Medical, Clinical, Pharmaceutical, Engineering, Technology and Management Fields.
Meet Inspiring Speakers and Experts at our 3000+ Global Conferenceseries Events with over 600+ Conferences, 1200+ Symposiums and 1200+ Workshops on Medical, Pharma, Engineering, Science, Technology and Business

Research Article Open Access

A Computational Model for Reconstructing Motor Commands by Analysis of Theoretically Generated Electromyograms

Keywords
Motor command; Action potential; Electromyography; Computer simulation
Abbreviations
EMG: Electromyography; IEMG: Integrated EMG; RMS: Root Mean Square
Introduction
In the process of controlling muscle activation, a motor command, which controls certain contraction level in a muscle, is generated in the motor area and transmitted through each motor neuron as a neural pulse train of action potentials [1]. The changes of the pulse density with neural recruitment correspond to the levels of motor commands. The action potentials from motor neurons are transmitted to muscle through end-plates and propagated to both tendons. Electromyography (EMG), which records the action potentials of a muscle, has been used in research and clinical applications of muscle activation.
In this study, we proposed the methods to reconstruct the motor commands by means of computation of detected pulse density from the EMG and validated the accuracy of reconstructing the motor commands with computer simulation.
The principal of reconstructing motor commands
The change of the pulse density in each of the motor neurons, if superimposed over a whole muscle, corresponds to the time pattern of the motor commands smoothened by transmission characteristics in the neural passage.
While muscle fibers connected to each motor neuron contract, the action potentials propagate along the muscle fibers. The bipolar surface electrodes for recording muscle activation are typically placed along the axis of the muscle fibers. The action potentials of the muscle fibers have tri-phasic pulses and are recorded with the electrodes as an EMG signal [2,3]. The time pattern of the motor commands is reconstructed by measuring time change in pulse density, after correcting the difference in amplitude among pulse trains caused by attenuation due to the distance from the motor neurons to the electrodes or the size of the motor unit.
A strategy for assessing accuracy of estimating motor command
Integrated EMG (IEMG) or root mean square (RMS) of EMG signals for computing the amplitude has been used widely to estimate the muscle activation or force level [4-6]. IEMG and RMS can be simply computed with the values of the EMG data. However, it is well known that the relationship between the force and the amplitude of EMG data could not be fitted by a single linear function in a high force level [7]. Validation would also be needed so that the result of reconstructing the motor command by data processing holds reasonable accuracy, even when the contraction of a muscle is very strong and the pulse density is very high because multiple action potentials would interfere with each other, making it difficult to identify the pulses from the EMG signal.
In order to assess the accuracy of the processing, a strategy using a computer simulation of estimating motor command is proposed. Between the processes of generation of neural pulses and observation of EMG, there are two signals, namely, the motor commands and the density modulated pulse trains for a muscle, which correspond each other.
If real EMG is used for data, it is not possible to observe explicitly the time pattern of the motor commands and the density modulated pulse trains. However, if all the steps involved in the processes of generating neural pulses in the motor neurons and observation of EMG are simulated in a computer, by introducing the neurophysiological properties as real as possible, the known generated motor commands and neural pulses serve as the reference for the detailed analysis of the nature of errors in the result obtained by the processing [8].
Methods
Procedure of generation of EMG
Following the sequence of the computer simulation program, neural pulse trains in each of the motor neurons before and after synapse were generated from motor command and the waveform of bipolar electrode EMG was synthesized (Figure 1). The characteristics of neural transmission were set by referring to the physiological data, and the number of motor units was chosen to be large enough for representing the motor commands of a muscle. A tri-phasic waveform of the action potential (Figure 2) was used for signal synthesis. The process of simulation consisted of the specification of the time pattern of the motor command, generation of pulse train in the motor neurons with low and high sensitivities, synthesis of EMG waveform from the near and far motor units with different attenuation (Figure 1d and 1e), detection of pulse train, and reconstruction of the time pattern of the motor command (Figure 1g).
Then, the pulse trains were detected from the EMG signal by pulse shaping, and the motor command of a muscle was reconstructed by measuring change in pulse density. Each time when a pulse was detected, the whole typical tri-phasic waveform of an action potential was subtracted from the EMG waveform. The sizes of motor units were considered as the same in this study.
The computer simulation program for the generation of EMG and reconstructing motor commands were executed as follows:
1. Specification of the time pattern of the motor command for a muscle.
2. Assignment of average characteristics of motor neurons before synapse and their random fluctuation.
a) Sensitivity of pulse generation in each pulse generator.
b) Refractory period in each pulse generator.
3. Generation of neural pulse train in each motor neuron before synapse.
4. Assignment of average characteristics of motor neuron after synapse and their random fluctuation.
a) Delay time of pulse regeneration at each synapse.
b) Refractory period at each synapse.
5. Generation of neural pulse train in each motor neuron after synapse.
6. Synthesis of the EMG waveform.
a) Assignment of the waveform of tri-phasic action potential.
b) Assignment of location of the motor units around the electrodes.
c) Computation of the interfered waveform by bipolar electrodes.
d) Synthesis of the EMG waveform.
7. Detection of the pulse trains from the EMG waveform by pulse shaping.
a) Set thresholds of the period and amplitude of the peaks of the pulses.
b) Register the time point of the pulse, which is larger than the threshold.
8. Reconstruction of the time pattern of the motor command by measuring pulse density.
9. Generation of the theoretical time pattern of the motor command.
The methods for detection of the pulse trains from the EMG waveform were the same methods used to investigate optimum electrode locations, far from innervation zone, for recording surface EMG signal by detected pulse-averaging as in the former report [9].
The software used in the present study was developed using QuickBASIC 1.0 (Microsoft) and the sampling rate for computer simulation and synthesis of EMG waveform was set to 8,000 Hz.
Results
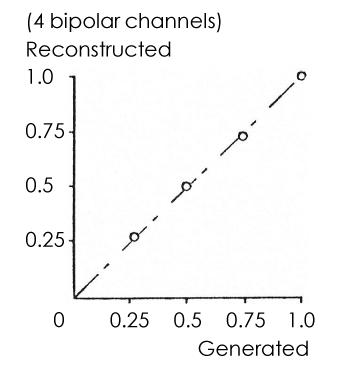
It was shown that the reconstructed time pattern of the motor command by means of these methods corresponded fairly accurately to the theoretically generated one, except for a few missing pulses in the motor units far from the electrodes, and a few false pulses caused by interference between high density pulse trains (Figure 3).
Discussion
The results of this study indicate that these proposed methods provide a direct approach to assessing the accuracy of reconstructing the motor commands. A few studies previously suggested that the motor commands were estimated from the surface EMG signal with the amplitude or specially designed multi-channel electrodes [10,11]. In this study, the processes of reconstruction of the neural pulse train and attenuation of EMG signal by the distance between the motor neurons and the usual bipolar electrodes were included in this simulation for the practical reconstructing of motor commands.
The ratio of detecting correct pulses would saturate at a value less than 100%, because closely located pairs of positive and negative pulses cancel each other in the theoretical EMG signal. In this study, the ratio of detecting false pulses increases when the third phase of the waveform of action potentials from a smaller amplitude pulse train was detected. However, the process of computer simulation could be modified in any complex way, if necessary for further analysis.
An action potential waveform
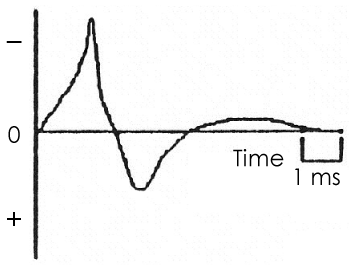
The typical action potential signal has been simulated with an EMG signal recorded using a pair of bipolar electrodes on the skin surface [12,13]. The calculated action potential is tri-phasic: the first and second phases and the amplitude of the low third phase amounts to 5-10% of the peak-to-peak amplitude. The first phase (or the second phase) has either a positive or a negative peak depending on the conduction direction [14]. In this study, the several sizes of tri-phasic waveforms were set to synthesize a theoretical EMG waveform.
Application for a real surface EMG signal
These proposed methods could be used for real EMG. However, several factors would have to be considered more for the actual application. The noises, which could be detected incorrectly as a pulse of action potential, are included in the real EMG signal. In this study, the EMG signal was synthesized under the assumption that the bipolar surface electrodes were placed along the axis of muscle fibers. However, the arrangement of muscle fibers can differ, depending on the anatomical structure of the muscle, which affects the EMG signal and appears to limit surface EMG analysis at this time [9,15].
Cross-talk, which involves signals generated from muscles other than the target muscle, must be minimized for accurate analysis. Double differentiation through the use of multiple electrodes is a general technique for reducing cross-talk [16,17].
Conclusions
The computer simulation proposed in this strategy has been executed preliminarily, and a promising result is suggested in this present study. Based on these results, it becomes possible to further assess the accuracy of these methods when reflecting neural pulses from the sensors in the muscle are introduced.
References

Visit for more related articles at Journal of Novel Physiotherapies

View PDF Download PDF Tables & Figures

Abstract

The methods to reconstruct motor commands, which control muscle contraction levels, by means of the computation of detected pulse density from a electromyography (EMG) signal was proposed. Moreover, the accuracy of reconstructing the motor commands with computer simulation was validated. Theoretical neural pulse trains in each of the motor neurons before and after synapse were generated from the specified time pattern of motor command for a muscle and the waveform of bipolar electrode EMG was synthesized. A tri-phasic waveform of the action potential was used for the synthesis. The locations of the motor units around the electrodes were assigned and the amplitude of the neural pulse trains in action potentials were attenuated according to the distances between the motor units and the electrodes. Then, pulse trains were detected from the synthesized EMG signal by pulse shaping, and the motor command of a whole muscle was reconstructed by measuring change in pulse density. The results showed that the reconstructed time pattern of the motor command corresponded fairly accurately to the theoretically generated one, except for a few missing pulses in the motor units far from the electrodes, and a few false pulses caused by interference between high density pulse trains. A direct approach to assessing the accuracy of reconstructing the motor commands was suggested with these methods.

Keywords
Motor command; Action potential; Electromyography; Computer simulation
Abbreviations
EMG: Electromyography; IEMG: Integrated EMG; RMS: Root Mean Square
Introduction
In the process of controlling muscle activation, a motor command, which controls certain contraction level in a muscle, is generated in the motor area and transmitted through each motor neuron as a neural pulse train of action potentials [1]. The changes of the pulse density with neural recruitment correspond to the levels of motor commands. The action potentials from motor neurons are transmitted to muscle through end-plates and propagated to both tendons. Electromyography (EMG), which records the action potentials of a muscle, has been used in research and clinical applications of muscle activation.
In this study, we proposed the methods to reconstruct the motor commands by means of computation of detected pulse density from the EMG and validated the accuracy of reconstructing the motor commands with computer simulation.
The principal of reconstructing motor commands
The change of the pulse density in each of the motor neurons, if superimposed over a whole muscle, corresponds to the time pattern of the motor commands smoothened by transmission characteristics in the neural passage.
While muscle fibers connected to each motor neuron contract, the action potentials propagate along the muscle fibers. The bipolar surface electrodes for recording muscle activation are typically placed along the axis of the muscle fibers. The action potentials of the muscle fibers have tri-phasic pulses and are recorded with the electrodes as an EMG signal [2,3]. The time pattern of the motor commands is reconstructed by measuring time change in pulse density, after correcting the difference in amplitude among pulse trains caused by attenuation due to the distance from the motor neurons to the electrodes or the size of the motor unit.
A strategy for assessing accuracy of estimating motor command
Integrated EMG (IEMG) or root mean square (RMS) of EMG signals for computing the amplitude has been used widely to estimate the muscle activation or force level [4-6]. IEMG and RMS can be simply computed with the values of the EMG data. However, it is well known that the relationship between the force and the amplitude of EMG data could not be fitted by a single linear function in a high force level [7]. Validation would also be needed so that the result of reconstructing the motor command by data processing holds reasonable accuracy, even when the contraction of a muscle is very strong and the pulse density is very high because multiple action potentials would interfere with each other, making it difficult to identify the pulses from the EMG signal.
In order to assess the accuracy of the processing, a strategy using a computer simulation of estimating motor command is proposed. Between the processes of generation of neural pulses and observation of EMG, there are two signals, namely, the motor commands and the density modulated pulse trains for a muscle, which correspond each other.
If real EMG is used for data, it is not possible to observe explicitly the time pattern of the motor commands and the density modulated pulse trains. However, if all the steps involved in the processes of generating neural pulses in the motor neurons and observation of EMG are simulated in a computer, by introducing the neurophysiological properties as real as possible, the known generated motor commands and neural pulses serve as the reference for the detailed analysis of the nature of errors in the result obtained by the processing [8].
Methods
Procedure of generation of EMG
Following the sequence of the computer simulation program, neural pulse trains in each of the motor neurons before and after synapse were generated from motor command and the waveform of bipolar electrode EMG was synthesized (Figure 1). The characteristics of neural transmission were set by referring to the physiological data, and the number of motor units was chosen to be large enough for representing the motor commands of a muscle. A tri-phasic waveform of the action potential (Figure 2) was used for signal synthesis. The process of simulation consisted of the specification of the time pattern of the motor command, generation of pulse train in the motor neurons with low and high sensitivities, synthesis of EMG waveform from the near and far motor units with different attenuation (Figure 1d and 1e), detection of pulse train, and reconstruction of the time pattern of the motor command (Figure 1g).
Then, the pulse trains were detected from the EMG signal by pulse shaping, and the motor command of a muscle was reconstructed by measuring change in pulse density. Each time when a pulse was detected, the whole typical tri-phasic waveform of an action potential was subtracted from the EMG waveform. The sizes of motor units were considered as the same in this study.
The computer simulation program for the generation of EMG and reconstructing motor commands were executed as follows:
1. Specification of the time pattern of the motor command for a muscle.
2. Assignment of average characteristics of motor neurons before synapse and their random fluctuation.
a) Sensitivity of pulse generation in each pulse generator.
b) Refractory period in each pulse generator.
3. Generation of neural pulse train in each motor neuron before synapse.
4. Assignment of average characteristics of motor neuron after synapse and their random fluctuation.
a) Delay time of pulse regeneration at each synapse.
b) Refractory period at each synapse.
5. Generation of neural pulse train in each motor neuron after synapse.
6. Synthesis of the EMG waveform.
a) Assignment of the waveform of tri-phasic action potential.
b) Assignment of location of the motor units around the electrodes.
c) Computation of the interfered waveform by bipolar electrodes.
d) Synthesis of the EMG waveform.
7. Detection of the pulse trains from the EMG waveform by pulse shaping.
a) Set thresholds of the period and amplitude of the peaks of the pulses.
b) Register the time point of the pulse, which is larger than the threshold.
8. Reconstruction of the time pattern of the motor command by measuring pulse density.
9. Generation of the theoretical time pattern of the motor command.
The methods for detection of the pulse trains from the EMG waveform were the same methods used to investigate optimum electrode locations, far from innervation zone, for recording surface EMG signal by detected pulse-averaging as in the former report [9].
The software used in the present study was developed using QuickBASIC 1.0 (Microsoft) and the sampling rate for computer simulation and synthesis of EMG waveform was set to 8,000 Hz.
Results
It was shown that the reconstructed time pattern of the motor command by means of these methods corresponded fairly accurately to the theoretically generated one, except for a few missing pulses in the motor units far from the electrodes, and a few false pulses caused by interference between high density pulse trains (Figure 3).
Discussion
The results of this study indicate that these proposed methods provide a direct approach to assessing the accuracy of reconstructing the motor commands. A few studies previously suggested that the motor commands were estimated from the surface EMG signal with the amplitude or specially designed multi-channel electrodes [10,11]. In this study, the processes of reconstruction of the neural pulse train and attenuation of EMG signal by the distance between the motor neurons and the usual bipolar electrodes were included in this simulation for the practical reconstructing of motor commands.
The ratio of detecting correct pulses would saturate at a value less than 100%, because closely located pairs of positive and negative pulses cancel each other in the theoretical EMG signal. In this study, the ratio of detecting false pulses increases when the third phase of the waveform of action potentials from a smaller amplitude pulse train was detected. However, the process of computer simulation could be modified in any complex way, if necessary for further analysis.
An action potential waveform
The typical action potential signal has been simulated with an EMG signal recorded using a pair of bipolar electrodes on the skin surface [12,13]. The calculated action potential is tri-phasic: the first and second phases and the amplitude of the low third phase amounts to 5-10% of the peak-to-peak amplitude. The first phase (or the second phase) has either a positive or a negative peak depending on the conduction direction [14]. In this study, the several sizes of tri-phasic waveforms were set to synthesize a theoretical EMG waveform.
Application for a real surface EMG signal
These proposed methods could be used for real EMG. However, several factors would have to be considered more for the actual application. The noises, which could be detected incorrectly as a pulse of action potential, are included in the real EMG signal. In this study, the EMG signal was synthesized under the assumption that the bipolar surface electrodes were placed along the axis of muscle fibers. However, the arrangement of muscle fibers can differ, depending on the anatomical structure of the muscle, which affects the EMG signal and appears to limit surface EMG analysis at this time [9,15].
Cross-talk, which involves signals generated from muscles other than the target muscle, must be minimized for accurate analysis. Double differentiation through the use of multiple electrodes is a general technique for reducing cross-talk [16,17].
Conclusions
The computer simulation proposed in this strategy has been executed preliminarily, and a promising result is suggested in this present study. Based on these results, it becomes possible to further assess the accuracy of these methods when reflecting neural pulses from the sensors in the muscle are introduced.

References
--

Figures at a glance

Figure Figure Figure
Figure 1 Figure 2 Figure 3
A Computational Model for Reconstructing Motor Commands by Analysis of Theoretically Generated Electromyograms
Post your comment

Share This Article

Relevant Topics

Recommended Journals

View More

Article Tools

Article Usage

  • Total views: 13039
  • [From(publication date):
    specialissue-2014 - Dec 27, 2024]
  • Breakdown by view type
  • HTML page views : 8707
  • PDF downloads : 4332

Post your comment

captcha   Reload  Can't read the image? click here to refresh
Peer Reviewed Journals

Make the best use of Scientific Research and information from our 700 + peer reviewed, Open Access Journals

Journals by Subject

Agri and Aquaculture Biochemistry Bioinformatics & Systems Biology Biomedical Sciences Business & Management Chemical Engineering Chemistry Clinical Sciences Computer Science Economics & Accounting Engineering Environmental Sciences Food & Nutrition General Science Genetics & Molecular Biology
Geology & Earth Science Immunology & Microbiology Informatics Materials Science Mathematics Medical Sciences Nanotechnology Neuroscience & Psychology Nursing & Health Care Pharmaceutical Sciences Physics Plant Sciences Social & Political Sciences Veterinary Sciences

Clinical & Medical Journals

Anesthesiology Cardiology Clinical Research Dentistry Dermatology Diabetes & Endocrinology Gasteroenterology Genetics Haematology Healthcare Immunology Infectious Diseases Medicine Microbiology
Molecular Biology Nephrology Neurology Nursing Nutrition Oncology Ophthalmology Orthopaedics Pathology Pediatrics Physicaltherapy & Rehabilitation
Psychiatry Pulmonology Radiology Reproductive Medicine Surgery Toxicology
International Conferences 2024-25
 
Meet Inspiring Speakers and Experts at our 3000+ Global Annual Meetings

Conferences by Country

USA Australia Italy Germany UK Japan Brazil South Korea Netherlands
Spain Canada China France India Singapore South Africa New Zealand Philippines
Poland Austria Finland Denmark Mexico Norway Romania

Medical & Clinical Conferences

Microbiology Diabetes & Endocrinology Nursing Healthcare Management Neuroscience Immunology Gastroenterology Genetics & MolecularBiology Pathology Alternative Healthcare Pediatrics Ophthalmology
Oncology & Cancer Cardiology Dentistry Physical Therapy Rehabilitation Psychiatry Infectious Diseases Medical Ethics & Health Policies Palliativecare Reproductive Medicine & Women Healthcare Surgery Radiology

Conferences By Subject

Pharmaceutical Sciences Pharma Marketing & Industry Agri, Food & Aqua Nutrition Physics & Materials Science Environmental Science EEE & Engineering Veterinary Chemical Engineering Business Management Massmedia Geology & Earth science
Top